Page 153 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

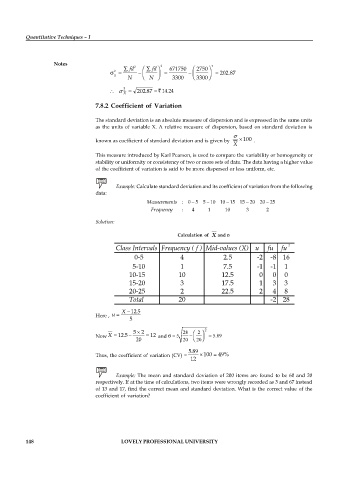
Notes 2 2
fd 2 fd 671750 2750
2 202 .87
X
N N 3300 3300
2
.
X 202 87 = 14.24
7.8.2 Coefficient of Variation

The standard deviation is an absolute measure of dispersion and is expressed in the same units
as the units of variable X. A relative measure of dispersion, based on standard deviation is

known as coefficient of standard deviation and is given by 100 .
X

This measure introduced by Karl Pearson, is used to compare the variability or homogeneity or
stability or uniformity or consistency of two or more sets of data. The data having a higher value
of the coefficient of variation is said to be more dispersed or less uniform, etc.

Example: Calculate standard deviation and its coefficient of variation from the following
data:
Measurements : 0 5 5 10 10 15 15 20 20 25
Frequency : 4 1 10 3 2
Solution:

Calculation of X and

Class Intervals Frequency ( f ) Mid-values (X) u fu fu 2
0-5 4 2.5 -2 -8 16
5-10 1 7.5 -1 -1 1
10-15 10 12.5 0 0 0
15-20 3 17.5 1 3 3
20-25 2 22.5 2 4 8
Total 20 -2 28

.
X 12 5
Here , u
5
5 2 28 2 2
Now X 12 5 12 and 5 5.89
.
20 20 20
.
5 89
Thus, the coefficient of variation (CV) = 100 49%.
12

Example: The mean and standard deviation of 200 items are found to be 60 and 20
respectively. If at the time of calculations, two items were wrongly recorded as 3 and 67 instead
of 13 and 17, find the correct mean and standard deviation. What is the correct value of the
coefficient of variation?

148 LOVELY PROFESSIONAL UNIVERSITY