Page 211 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes Nature of weights

While taking weighted average of price relatives, the values are often taken as weights. These
weights can be the values of base year quantities valued at base year prices, i.e., p q , or the
0i 0i
values of current year quantities valued at current year prices, i.e., p q , or the values of current
1i 1i
year quantities valued at base year prices, i.e., p q , etc., or any other value.
0i 1i
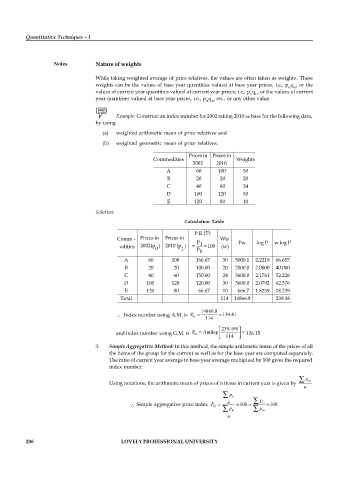
Example: Construct an index number for 2002 taking 2010 as base for the following data,
by using

(a) weighted arithmetic mean of price relatives and
(b) weighted geometric mean of price relatives.

Prices in Prices in
Commodities Weights
2002 2010
A 60 100 30
B 20 20 20
C 40 60 24
D 100 120 30
E 120 80 10
Solution:
Calculation Table

P.R. P
Comm - Prices in Prices in Wts
p Pw log P w log P
odities 2002(p ) 2010 (p ) = 1 ×100 (w)
0 1 p
0
A 60 100 166.67 30 5000.1 2.2219 66.657
B 20 20 100.00 20 2000.0 2.0000 40.000
C 40 60 150.00 24 3600.0 2.1761 52.226
D 100 120 120.00 30 3600.0 2.0792 62.376
E 120 80 66.67 10 666.7 1.8239 18.239
Total 114 14866.8 239.48

14866.8
Index number using A.M. is P 01 114 130.41

239.498
and index number using G.M. is P 01 Antilog 126.15
114
3. Simple Aggregative Method: In this method, the simple arithmetic mean of the prices of all
the items of the group for the current as well as for the base year are computed separately.
The ratio of current year average to base year average multiplied by 100 gives the required
index number.

p
Using notations, the arithmetic mean of prices of n items in current year is given by 0i
n
p
1i
n p 1i
Simple aggregative price index P 100 100
01
p p
0i 0i
n

206 LOVELY PROFESSIONAL UNIVERSITY