Page 313 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I
P. 313
Quantitative Techniques – I
Notes The fitting of a normal curve can be done by
(a) The Method of Ordinates or
(b) The Method of Areas.
(a) Method of Ordinates: In this method, the ordinate f(X) of the normal curve, for various
values of the random variate X are obtained by using the table of ordinates for a
standard normal variate.
2
1 X 1
1 2 1 z 2 1
We can write f X e e 2 z
2 2
1 2
X 1 z
where z and z e 2 .
2
The expected frequency corresponding to a particular value of X is given by
N
y N.f X z and therefore, the expected frequency of a class = y h, where h
is the class interval.
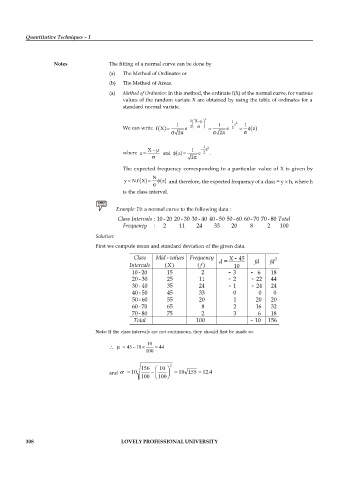
Example: Fit a normal curve to the following data :
Class Intervals : 10 - 20 20 - 30 30 - 40 40 - 50 50 - 60 60 -70 70 - 80 Total
Frequency : 2 11 24 33 20 8 2 100
Solution:
First we compute mean and standard deviation of the given data.
Class Mid - values Frequency X - 45 2
d = fd fd
Intervals (X) ( f ) 10
10 - 20 15 2 - 3 - 6 18
20 - 30 25 11 - 2 - 22 44
30 - 40 35 24 - 1 - 24 24
40 - 50 45 33 0 0 0
50 - 60 55 20 1 20 20
60 -70 65 8 2 16 32
70 - 80 75 2 3 6 18
Total 100 - 10 156
Note: If the class intervals are not continuous, they should first be made so.
10
45 10 44
100
2
156 10
.
.
and 10 10 155 12 4
100 100
308 LOVELY PROFESSIONAL UNIVERSITY