Page 55 - DECO101_MICRO_ECONOMICS_ENGLISH
P. 55
Micro Economics
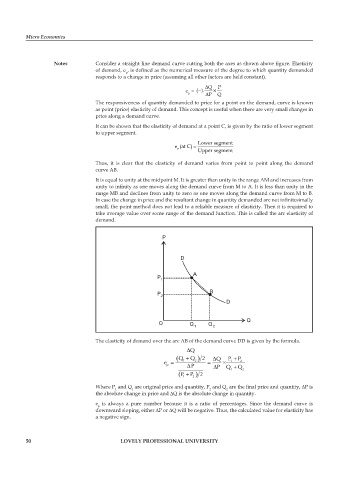
Notes Consider a straight line demand curve cutting both the axes as shown above fi gure. Elasticity
of demand, e , is defi ned as the numerical measure of the degree to which quantity demanded
p
responds to a change in price (assuming all other factors are held constant).
Δ Q P
−
e = (). ×
p Δ P Q
The responsiveness of quantity demanded to price for a point on the demand, curve is known
as point (price) elasticity of demand. This concept is useful when there are very small changes in
price along a demand curve.
It can be shown that the elasticity of demand at a point C, is given by the ratio of lower segment
to upper segment.
Lower segment
e(at C) =
p
Upper segment
Thus, it is clear that the elasticity of demand varies from point to point along the demand
curve AB.
It is equal to unity at the midpoint M. It is greater than unity in the range AM and increases from
unity to infinity as one moves along the demand curve from M to A. It is less than unity in the
range MB and declines from unity to zero as one moves along the demand curve from M to B.
In case the change in price and the resultant change in quantity demanded are not infi nitesimally
small, the point method does not lead to a reliable measure of elasticity. Then it is required to
take average value over some range of the demand function. This is called the arc elasticity of
demand.
P
D
A
P 1
P 2 B
D
O Q 1 Q 2 Q
The elasticity of demand over the arc AB of the demand curve DD is given by the formula.
ΔQ
(Q + Q ) 2 ΔQ P + P
e p = 1 ΔP 2 = ΔP × Q 1 + Q 2
( +P 1 P 2 ) 2 1 2
Where P and Q are original price and quantity, P and Q are the final price and quantity, ∆P is
1 1 2 2
the absolute change in price and ∆Q is the absolute change in quantity.
e is always a pure number because it is a ratio of percentages. Since the demand curve is
p
downward sloping, either ∆P or ∆Q will be negative. Thus, the calculated value for elasticity has
a negative sign.
50 LOVELY PROFESSIONAL UNIVERSITY