Page 105 - DECO403_MATHEMATICS_FOR_ECONOMISTS_ENGLISH

Mathematics for Economists

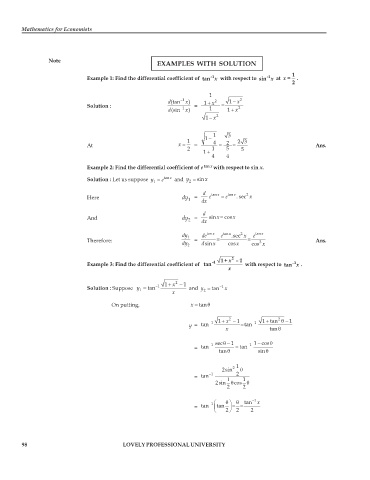
Note
EXAMPLES WITH SOLUTION
1
–1
–1
Example 1: Find the differential coefficient of tan x with respect to sin x at =x .
2
1
1
d (tan x ) 1 x 2 1 x 2
Solution : =
1
d (sin x ) 1 1 x 2
1 x 2
1 3
1 1 4 23
At x = 2 Ans.
2 1 5 5
1
4 4
Example 2: Find the differential coefficient of e tan x with respect to sin x.
Solution : Let us suppose y 1 e tan x and y 2 sinx

d tan x tan x 2
Here dy 1 = dx e e .sec x

d
And dy 2 = dx sinx cosx

2
dy 1 de tan x e tan x .sec x e tan x
Therefore: dy 2 = d sinx cosx cos x Ans.
3
–1 1+ x 2 – 1
Example 3: Find the differential coefficient of tan with respect to tan x .
–1
x
1 1 x 2 1

Solution : Suppose y 1 tan and y tan 1 x
x 2
On putting, tanx T

2 1 1 1 x 1 2 T tan 1

y = tan tan 1
x tan T
T 1 1 sec 1 1cos T

= tan tan T tan sin T

2 1
2sin T
= tan 1 2
T 2sin 1 Tcos
2 2

1
T ·
= tan § 1 ¨ tan T ¸ tan x
© 2 ¹ 2 2

98 LOVELY PROFESSIONAL UNIVERSITY