Page 113 - DECO403_MATHEMATICS_FOR_ECONOMISTS_ENGLISH

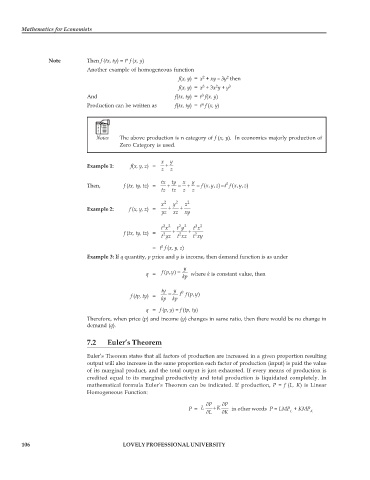
Mathematics for Economists

n
Note Then f (tx, ty) = t f (x, y)
Another example of homogeneous function
2
f(x, y)= x + xy – 3y then
2
f(x, y)= x + 3x y + y 3
3
2
3
And f(tx, ty)= t f(x, y)
n
Production can be written as f(tx, ty)= t f (x, y)

Notes The above production is n category of f (x, y). In economics majorly production of
Zero Category is used.

x y
Example 1: f(x, y, z)=
z z
tx ty x y
0
z
Then, f (tx, ty, tz)= f x y (, , ) z t f (, , )
y
x
tz tz z z
x 2 y 2 z 2
Example 2: f (x, y, z)=
yz xz xy
22
2 2
22
tx ty t z
f (tx, ty, tz)= tyz t xz t xy
2
2
2
0
= t f (x, y, z)
Example 3: If q quantity, p price and y is income, then demand function is as under
y
(, )
q = fp y kp where M is constant value, then
ty y 0
y
p
f (tp, ty)= kp kp tf (, )
q = f (p, y) = f (tp, ty)
Therefore, when price (p) and income (y) changes in same ratio, then there would be no change in
demand (q).

7.2 Euler’s Theorem

Euler’s Theorem states that all factors of production are increased in a given proportion resulting
output will also increase in the same proportion each factor of production (input) is paid the value
of its marginal product, and the total output is just exhausted. If every means of production is
credited equal to its marginal productivity and total production is liquidated completely. In
mathematical formula Euler’s Theorem can be indicated. If production, P = f (L, K) is Linear
Homogeneous Function:

w wP P
P = L K in other words P = LMP + KMP
w wL K L K

106 LOVELY PROFESSIONAL UNIVERSITY