Page 40 - DECO403_MATHEMATICS_FOR_ECONOMISTS_ENGLISH

Unit 2: Limits and Continuity

Then A is called limit of f(x) at x = a Note
Set h , h ,......, h ,...... is a sequence, for which limit is 0. Similarly second makes sequence (2). Here is
2
n
1
it to be specially noted that for limit to exist, like sequence (1) f(a + h ) every type of sequence should
n
tend to A. viz the statistical difference of f(a – h ) – A, choosing h sufficient smaller, can be reduced
n
n
as desired. Assigning a + h {or a – h } = x or | x – a | = h we can define the limit as under
n n n
Definition – At x = a, limit of function f(x) is any number (assume A, which has the property that for
each value of x for which |x – a| viz x – a is numerical value) sufficiently smaller (but not zero), |f(x)
– A| viz the numerical value of f(x)-A is smaller as desired.
Limit can also be defined with the following
Second definition of limit
When x →→ →→ → a (when x tends to a), the limit of function f(x) is any number (Assume A), which has the
property that for any independent positive smallest number ε, a second number δ greatest than 0 can
be obtained, for which| ()fx – A|< ε for every values of x,
0< | x – a | < δ.

Notes If at x = a, L is the limit (L) of f(x), then this can be expressed as

"

"

>;?;F = L or >;? = L.
" "
2.2 Right Hand and Left Hand Limits
2.2.1 Right Hand Limit

When the limit of function is obtained from the right hand of the independent variable, then it is
called Right Hand Limit (R.H.L.) and applying positive (+) sign for the right side, this can be
expressed as under
Right Hand Limit = f(a + 0) = lim ( )fx = l .
x o a 1

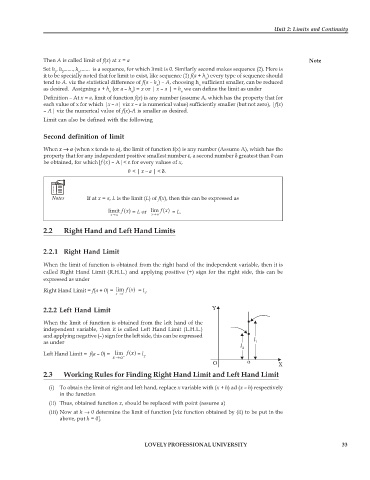
2.2.2 Left Hand Limit Y

When the limit of function is obtained from the left hand of the
independent variable, then it is called Left Hand Limit (L.H.L.)
and applying negative (–) sign for the left side, this can be expressed
as under l 1
l 2
( )
Left Hand Limit = f(a – 0) = lim fx = l .
x oa 2
O a X
2.3 Working Rules for Finding Right Hand Limit and Left Hand Limit

(i) To obtain the limit of right and left hand, replace x variable with (x + h) ad (x – h) respectively
in the function
(ii) Thus, obtained function x, should be replaced with point (assume a)
(iii) Now at h → 0 determine the limit of function [viz function obtained by (ii) to be put in the
above, put h = 0].

LOVELY PROFESSIONAL UNIVERSITY 33