Page 32 - DMTH401_REAL ANALYSIS

Real Analysis

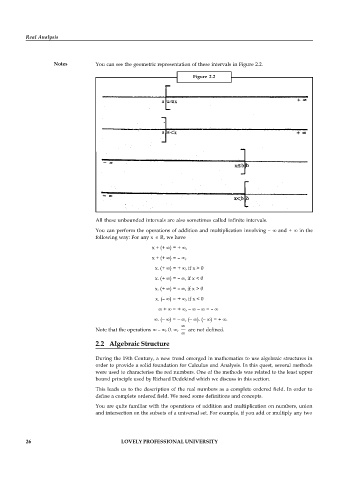
Notes You can see the geometric representation of these intervals in Figure 2.2.
Figure 2.2

All these unbounded intervals are also sometimes called infinite intervals.
You can perform the operations of addition and multiplication involving –  and +  in the
following way: For any x  R, we have
x + (+ ) = + ,
x + (+ ) = – ,
x. (+ ) = + , if x > 0
x. (+ ) = – , if x < 0

x. (+ ) = – , if x > 0
x. (– ) = + , if x < 0
 +  = + , –  –  = – 

. (– ) = – , (– ). (– ) = + .

Note that the operations  – , 0. , are not defined.

2.2 Algebraic Structure

During the 19th Century, a new trend emerged in mathematics to use algebraic structures in
order to provide a solid foundation for Calculus and Analysis. In this quest, several methods
were used to characterise the red numbers. One of the methods was related to the least upper
bound principle used by Richard Dedekind which we discuss in this section.
This leads us to the description of the real numbers as a complete ordered field. In order to
define a complete ordered field. We need some definitions and concepts.
You are quite familiar with the operations of addition and multiplication on numbers, union
and intersection on the subsets of a universal set. For example, if you add or multiply any two

26 LOVELY PROFESSIONAL UNIVERSITY