Page 15 - DMTH404_STATISTICS

Unit 1: Sample Space of A Random Experiment

Let  = { GGG, GGB, GBG, BGG, BBG, BGB, GBB, BBB } be the sample space correspondintog Notes
Experiment 5. Let A = {BBG, BGB, GBB} be the event that only one of the three inspected items is
good. Here the point BGB is an element of the set A and the point BBB is not an element of A. We
express this by writing BGB  A and BBB  A.

Notes A = {w  Q} | w  A}. Then  =  and  = . Fig. 1 shows a Venn diagram
c
c
c
c
representing the sets A and A .
Suppose, now, that the outcome of the experiment is BBB. Obviously, the event A has not
occurred. But, we may say the event “not A” hasaoccurred. In probability theory, the event “not
A” is called the event complementary to A and is denoted by A . c
Let’s try to understand this concept by looking back at Experiments 3-11.

Examples:
(i) For Experiment 5, if A = {BBG, BGB, GBB} , then
A = {GGG, GGB, BGG, GBG, BBB}.
C
(ii) In Experiment 6, let A denote the event that the number of infected persons is at most 40.
Then
A = {(x, y) | x + y > 40, x = 0, 1, ..., 30, y = 0, 1, ..., 20}.
c
(iii) In Experiment 11, if B denotes the event that none of the 13 cards is a spade, B consists of
c
all hands of 13 cards, each one of which has at least one spade.
Suppose now that A and A are two events associated with an experiment. We can get two new
1 2
events, A   A (A intersection A ) and A   A (A union A ) from these two. With your
l 2 1 2 1 2 1 2
knowledge of set theofy (MTE-04). you would expect the event A A to correspond to the set
1 2
whose elements belong to both A and A . Thus,
1 2
A A = { |   A and   A }.
1 2  2
Similarly, the event A  A corresponds to the set whose elements belong to at least one of
1 2
A and A .
1 2
A A = { |   A or   A }.
1 2 1 2
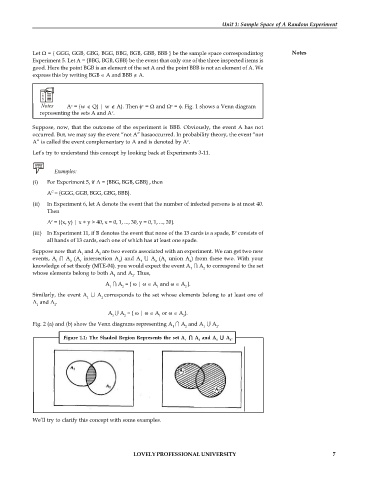
Fig. 2 (a) and (b) show the Venn diagrams representing A A and A A .
1 2 1 2
Figure 1.1: The Shaded Region Represents the set A   A and A   A .
1 2 1 2

We’ll try to clarify this concept with some examples.

LOVELY PROFESSIONAL UNIVERSITY 7