Page 69 - DMTH505_MEASURE_THEOREY_AND_FUNCTIONAL_ANALYSIS

Measure Theory and Functional Analysis

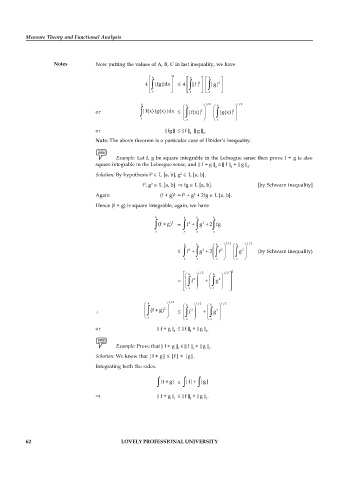
Notes Now putting the values of A, B, C in last inequality, we have
2
b b b
4 |fg|dx 4 |f| 2 |g| 2
a a a

b b 1/2 b 1/2
or |f(x) (g(x)|dx |f(x)| 2 |g(x)| 2
a
a a
or fg f g .
2 2
Note: The above theorem is a particular case of Hölder’s inequality.

Example: Let f, g be square integrable in the Lebesgue sense then prove f + g is also
square integrable in the Lebesgue sense, and f + g f + g .
2 2 2
2
2
Solution: By hypothesis f L [a, b], g L [a, b].
2
2
f , g L [a, b] fg L [a, b]. [by Schwarz inequality]
2
2
2
Again (f + g) = f + g + 2fg L [a, b].
Hence (f + g) is square integrable, again, we have
b b b b
(f g) 2 = f 2 g 2 2 fg
a a a a
1/2 1/2
b b b b
f 2 g 2 2 f 2 g 2 (by Schwarz inequality)
a a a a

1/2 1/2 2
b b
= f 2 g 2
a a

1/2
b 1/2 1/2
b b
(f g) 2 f 2 g 2
a
a a
or f + g f + g .
2 2 2
Example: Prove that f + g f + g .
1 1 1
Solution: We know that |f + g| |f| + |g|.
Integrating both the sides.

|f g| |f| |g|

f + g f + g .
1 1 1

62 LOVELY PROFESSIONAL UNIVERSITY