Page 243 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

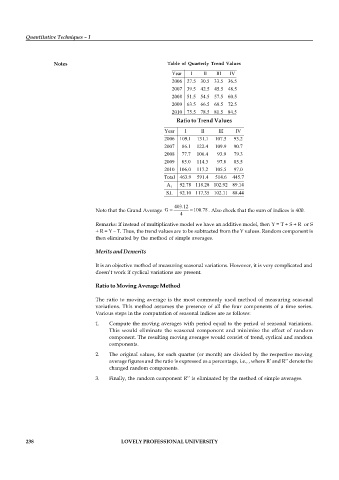
Notes Table of Quarterly Trend Values
Year I II III IV
2006 27.5 30.5 33.5 36.5
2007 39.5 42.5 45.5 48.5
2008 51.5 54.5 57.5 60.5
2009 63.5 66.5 69.5 72.5
2010 75.5 78.5 81.5 84.5
Ratio to Trend Values
Year I II III IV
2006 109.1 131.1 107.5 93.2
2007 86.1 122.4 109.9 90.7
2008 77.7 106.4 93.9 79.3
2009 85.0 114.3 97.8 85.5
2010 106.0 117.2 105.5 97.0
Total 463.9 591.4 514.6 445.7
A i 92.78 118.28 102.92 89.14
S.I. 92.10 117.35 102.11 88.44

403.12
Note that the Grand Average G 100.78 . Also check that the sum of indices is 400.
4
Remarks: If instead of multiplicative model we have an additive model, then Y = T + S + R or S
+ R = Y – T. Thus, the trend values are to be subtracted from the Y values. Random component is
then eliminated by the method of simple averages.

Merits and Demerits

It is an objective method of measuring seasonal variations. However, it is very complicated and
doesn’t work if cyclical variations are present.

Ratio to Moving Average Method

The ratio to moving average is the most commonly used method of measuring seasonal
variations. This method assumes the presence of all the four components of a time series.
Various steps in the computation of seasonal indices are as follows:
1. Compute the moving averages with period equal to the period of seasonal variations.
This would eliminate the seasonal component and minimise the effect of random
component. The resulting moving averages would consist of trend, cyclical and random
components.
2. The original values, for each quarter (or month) are divided by the respective moving
average figures and the ratio is expressed as a percentage, i.e., , where R’ and R’’ denote the
changed random components.
3. Finally, the random component R’’ is eliminated by the method of simple averages.

238 LOVELY PROFESSIONAL UNIVERSITY