Page 73 - DCAP108_DIGITAL_CIRCUITS_AND_LOGIC_DESIGNS

Digital Circuits and Logic Design

Notes Thus, the resultant expression for X is simply X = C. This can be proved as follows:
X = ABC + ABC + ABC + ABC
(
= AC B( + B) + AC B + B)
= AC + AC
= CA( + A) = C

As another example, consider Figure 4.19(d), where the four squares containing 1s are
ABCD ABCD ABCD, and ABCD. Examination of these terms indicates that only the variables
,
A and D remain unchanged, so that the simplified expression)r X is
X = AD
To summarize:

Looping a quad of 1s eliminates the two variables that appear in both complemented and
uncomplemented form.

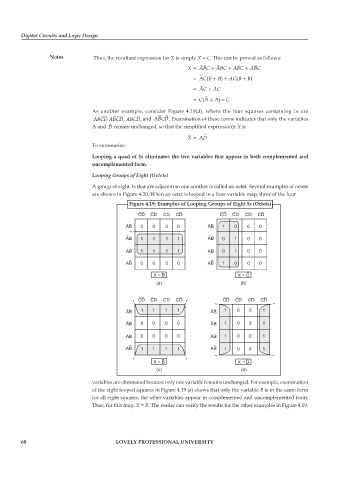
Looping Groups of Eight (Octets)
A group of eight 1s that are adjacent to one another is called an octet. Several examples of octets
are shown in Figure 4.20. When an octet is looped in a four-variable map, three of the four
Figure 4.19: Examples of Looping Groups of Eight 1s (Octets)

variables are eliminated because only one variable remains unchanged. For example, examination
of the eight looped squares in Figure 4.19 (a) shows that only the variable B is in the same form
for all eight squares; the other variables appear in complemented and uncomplemented form.
Thus, for this map, X = B. The reader can verify the results for the other examples in Figure 4.19.

68 LOVELY PROFESSIONAL UNIVERSITY