Page 10 - DMTH201_Basic Mathematics-1
P. 10
Unit 1: Trigonometric Functions-I
Now let the point P move from its original position in anti–clockwise direction. For various Notes
positions of this point in the four quadrants, various real numbers q will be generated. We
summarise, the above discussion as follows. For values of q in the:
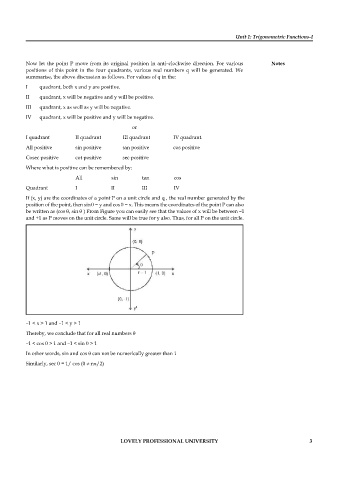
I quadrant, both x and y are positive.
II quadrant, x will be negative and y will be positive.
III quadrant, x as well as y will be negative.
IV quadrant, x will be positive and y will be negative.
or
I quadrant II quadrant III quadrant IV quadrant.
All positive sin positive tan positive cos positive
Cosec positive cot positive sec positive
Where what is positive can be remembered by:
All sin tan cos
Quadrant I II III IV
If (x, y) are the coordinates of a point P on a unit circle and q , the real number generated by the
position of the point, then sin = y and cos = x. This means the coordinates of the point P can also
be written as (cos , sin ) From Figure you can easily see that the values of x will be between –1
and +1 as P moves on the unit circle. Same will be true for y also. Thus, for all P on the unit circle.
–1 < x > 1 and –1 < y > 1
Thereby, we conclude that for all real numbers
–1 < cos > 1 and –1 < sin > 1
In other words, sin and cos can not be numerically greater than 1
Similarly, sec = 1/ cos ( n /2)
LOVELY PROFESSIONAL UNIVERSITY 3