Page 126 - DMTH201_Basic Mathematics-1

Unit 4: Determinants

Notes
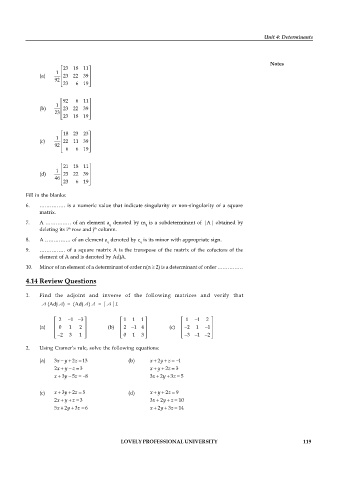
23 18 11
1
(a) 23 22 39
92
23 6 19

92 6 11
1
(b) 23 22 39
23
23 18 19

18 23 23
1
(c) 22 11 39
92
6 6 19

21 18 11
1
(d) 23 22 39
46
23 6 19
Fill in the blanks:

6. …………… is a numeric value that indicate singularity or non-singularity of a square
matrix.
7. A …………… of an element a denoted by m is a subdeterminant of |A| obtained by
ij ij
th
th
deleting its i row and j column.
8. A …………… of an element a denoted by c is its minor with appropriate sign.
ij ij
9. …………… of a square matrix A is the transpose of the matrix of the cofactors of the
element of A and is denoted by AdjA.
10. Minor of an element of a determinant of order n(n 2) is a determinant of order ……………

4.14 Review Questions

1. Find the adjoint and inverse of the following matrices and verify that
(Adj ) (Adj ) I.
A
A
A
A
A
2 1 3 1 1 1 1 1 2
(a) 0 1 2 (b) 2 1 4 (c) 2 1 1
2 3 1 0 1 3 3 1 2
2. Using Cramer’s rule, solve the following equations:

(a) 3x y 2z 13 (b) x 2y z 1
2x y z 3 x y 2z 3
x 3y 5z 8 3x 2y 3z 5

(c) x 3y 2z 5 (d) x y 2z 9
2x y z 3 3x 2y z 10
5x 2y 3z 6 x 2y 3z 14

LOVELY PROFESSIONAL UNIVERSITY 119