Page 150 - DMTH201_Basic Mathematics-1

Unit 5: Equations of Straight Lines

Notes
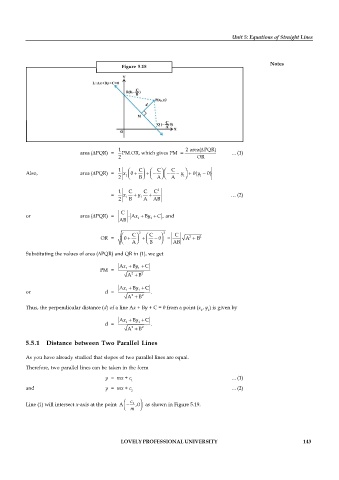
Figure 5.18

1 2 area( PQR)
area ( PQR) = PM.OR, which gives PM ... (1)
2 OR

1 C C C
Also, area ( PQR) = x 1 0 y 1 0(y 1 0)
2 B A A

1 C C C 2
= x 1 y 1 … (2)
2 B A AB
C
or area ( PQR) = Ax 1 By 1 C , and
AB

C 2 C 2 C
OR = 0 0 A 2 B 2
A B AB
Substituting the values of area ( PQR) and QR in (1), we get

Ax 1 By 1 C
PM =
A 2 B 2

Ax 1 By 1 C
or d = .
A 2 B 2
Thus, the perpendicular distance (d) of a line Ax + By + C = 0 from a point (x , y ) is given by
1 1
Ax 1 By 1 C
d = .
A 2 B 2

5.5.1 Distance between Two Parallel Lines

As you have already studied that slopes of two parallel lines are equal.
Therefore, two parallel lines can be taken in the form
y = mx + c ... (1)
1
and y = mx + c ... (2)
2
c
Line (1) will intersect x-axis at the point A 1 ,0 as shown in Figure 5.19.
m

LOVELY PROFESSIONAL UNIVERSITY 143