Page 204 - DMTH201_Basic Mathematics-1

Unit 7: Limits

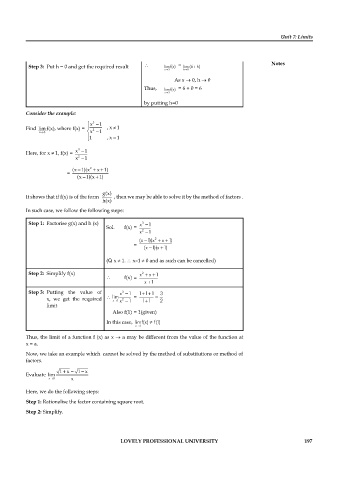
Step 3: Put h = 0 and get the required result = Notes

As x 0, h 0
Thus, = 6 + 0 = 6

by putting h=0
Consider the example:

Find , where f(x) =

Here, for x 1, f(x) =

=

It shows that if f(x) is of the form , then we may be able to solve it by the method of factors .

In such case, we follow the following steps:
Step 1: Factorise g(x) and h (x)
Sol. f(x) =

=

(Q x 1. x–1 0 and as such can be cancelled)

Step 2: Simplify f(x)
f(x) =

Step 3: Putting the value of
=
x, we get the required
limit
Also f(1) = 1(given)
In this case,

Thus, the limit of a function f (x) as x a may be different from the value of the function at
x = a.
Now, we take an example which cannot be solved by the method of substitutions or method of
factors.

Evaluate

Here, we do the following steps:

Step 1: Rationalise the factor containing square root.
Step 2: Simplify.

LOVELY PROFESSIONAL UNIVERSITY 197