Page 84 - DMTH201_Basic Mathematics-1
P. 84
Unit 4: Determinants
Notes
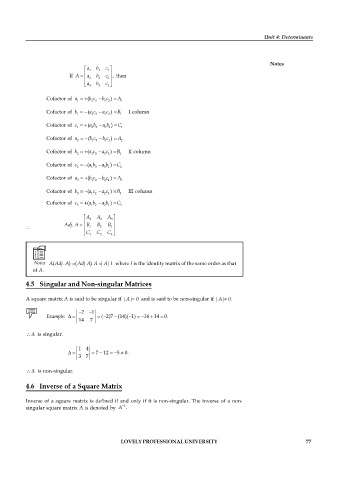
a b c
1 1 1
If A a 2 b 2 c 2 , then
a b c
3 3 3
Cofactor of a 1 (b c b c ) A 1
2 3
3 2
Cofactor of b 1 (a c a c ) B 1 I column
2 3
3 2
Cofactor of c (a b a b ) C
1 2 3 3 2 1
Cofactor of a (b c b c ) A
2 1 3 3 1 2
Cofactor of b 2 (a c a c ) B 2 II column
3 1
1 3
Cofactor of c 2 (a b a b ) C 2
3 1
1 3
Cofactor of a 3 (b c b c ) A 3
2 1
1 2
Cofactor of b (a c a c ) B III column
3 1 2 2 1 3
Cofactor of c 3 (a b a b ) C 3
2 1
1 2
A 1 A 2 A 3
Adj . A B B B
1 2 3
C C C
1 2 3
.
)
Notes (A Adj A ) (Adj A A | |I where I is the identity matrix of the same order as that
A
of A.
4.5 Singular and Non-singular Matrices
A square matrix A is said to be singular if | | 0A and is said to be non-singular if | | 0.A
2 1
Example: ( 2)7 (14)( 1) 14 14 0.
14 7
is singular.
1 4
7 12 5 0.
3 7
is non-singular.
4.6 Inverse of a Square Matrix
Inverse of a square matrix is defined if and only if it is non-singular. The inverse of a non-
singular square matrix A is denoted by A 1 .
LOVELY PROFESSIONAL UNIVERSITY 77