Page 97 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

Notes
Did u know? By means of a limit approach, and the knowledge that this outlines, or integral,
over a particular range in x is the area under the curve, A is the limit of the sum as Dx leads
to zero.

x 2
2
A  lim    x  0  x dx
x
 x 0 n
n
The integral is scrutinized as the area produced by summing an infinite number of rectangles of
infinitely minute width. The antiderivatives of these integrals can be revealed by the estimation
method sketched to be equal to the area under the curve. In definite practice the integrals are
frequently mentioned not as 0 to x but as from a lower limit to a higher limit corresponding to
the area preferred.
The next three examples demonstrate the utilization of integrals in locating the area of a rectangle,
triangle and area under a quadratic. These integrals exhibit that the area under the curve as
displayed by the integral is in fact the antiderivative with the specified limits. This is easily
confirmed by performing estimations as summarized above.

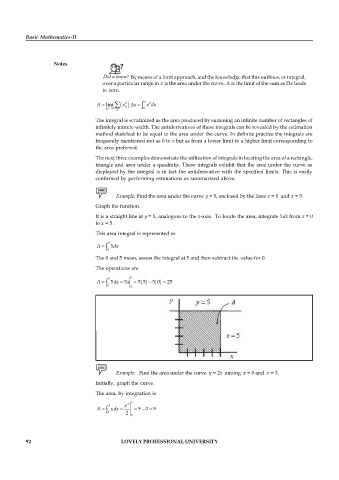
Example: Find the area under the curve y = 5, enclosed by the lines x = 0 and x = 5.

Graph the function.
It is a straight line at y = 5, analogous to the x-axis. To locate the area, integrate 5dx from x = 0
to x = 5 .

This area integral is represented as
5
A  0  5dx
The 0 and 5 mean, assess the integral at 5 and then subtract the value for 0.
The operations are
5
5
A  0  5dx  5x  5   5  5   0  25
0

Example: Find the area under the curve y = 2x among x = 0 and x = 3.

Initially, graph the curve.
The area, by integration is

3 x 2 3

A  0  xdx   9 0  9
2
0

92 LOVELY PROFESSIONAL UNIVERSITY