Page 130 - DMTH201_Basic Mathematics-1

Unit 5: Equations of Straight Lines

Introduction Notes

In this unit we find the equation of a straight line, when we are given some information about
the line. Straight-line equations, or "linear" equations, graph as straight lines, and have simple
variable expressions with no exponents on them.. The information could be the value of its
gradient, together with the co-ordinates of a point on the line. Alternatively, the information
might be the co-ordinates of two different points on the line. There are several different ways of
expressing the final equation, and some are more general than others. In order to master the
techniques explained here it is vital that you undertake plenty of practice exercises so that they
2
become second nature. If you see an equation with only x and y as opposed to, say x or sqrt(y)
- then you're dealing with a straight-line equation.

5.1 Distance between Two Points

As we know that coordinates are the pairs of numbers that defining the position of a point on a
two-dimensional plane. Given the coordinates of two points, the distance D between the points
is given by:
D = dx 2 dy 2

where dx is the difference between the x-coordinates of the points and dy is the difference
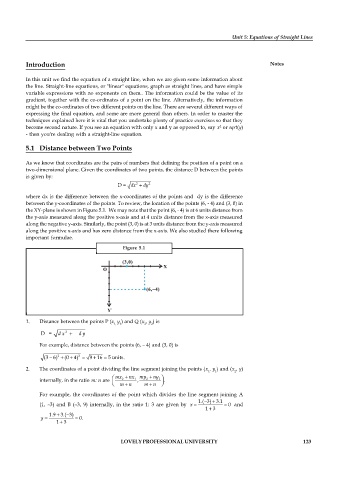
between the y-coordinates of the points. To review, the location of the points (6, - 4) and (3, 0) in
the XY-plane is shown in Figure 5.1. We may note that the point (6, - 4) is at 6 units distance from
the y-axis measured along the positive x-axis and at 4 units distance from the x-axis measured
along the negative y-axis. Similarly, the point (3, 0) is at 3 units distance from the y-axis measured
along the positive x-axis and has zero distance from the x-axis. We also studied there following
important formulae.
Figure 5.1

1. Distance between the points P (x y ) and Q (x , y ) is
1, 1 2 2
D = dx 2 dy 2
For example, distance between the points (6, – 4) and (3, 0) is

(3 6) 2 (0 4) 2 9 16 5 units.
2. The coordinates of a point dividing the line segment joining the points (x , y ) and (x , y)
1 1 2
mx nx my ny
internally, in the ratio m: n are 2 1 , 2 1 .
m n m n
For example, the coordinates of the point which divides the line segment joining A
1.( 3) 3.1
(1, –3) and B (–3, 9) internally, in the ratio 1: 3 are given by x 0 and
1 3
1.9 3.( 3)
y 0.
1 3

LOVELY PROFESSIONAL UNIVERSITY 123