Page 244 - DMTH201_Basic Mathematics-1

Unit 8: Continuity

Notes
In general if , where p(x) and q(x) are polynomial function and q(x) then f(x)
is continuous if p(x) and q(x) both are continuous.

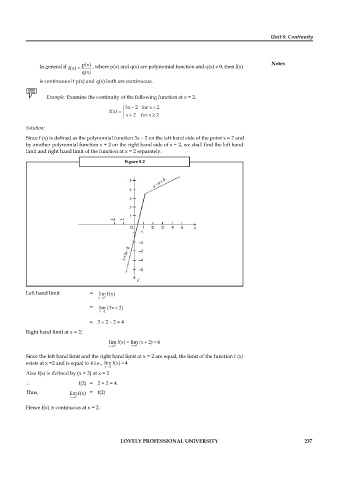
Example: Examine the continuity of the following function at x = 2.

Solution:
Since f (x) is defined as the polynomial function 3x – 2 on the left hand side of the point x = 2 and

by another polynomial function x + 2 on the right hand side of x = 2, we shall find the left hand

limit and right hand limit of the function at x = 2 separately.
Figure 8.2

Left hand limit =

=

= 3 2 – 2 = 4
Right hand limit at x = 2;

Since the left hand limit and the right hand limit at x = 2 are equal, the limit of the function f (x)
.
exists at x =2 and is equal to 4 i.e.,

Also f(x) is defined by (x + 2) at x = 2
f(2) = 2 + 2 = 4.
Thus, = f(2)

Hence f(x) is continuous at x = 2.

LOVELY PROFESSIONAL UNIVERSITY 237