Page 278 - DMTH201_Basic Mathematics-1
P. 278
Unit 10: Logarithmic Differentiation
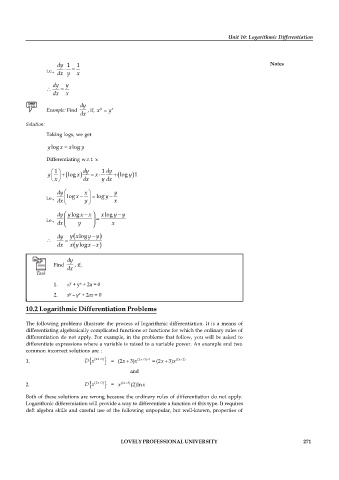
dy 1 1 Notes
i.e.,
dx y x
dy y
dx x
dy
Example: Find , if, x y y x
dx
Solution:
Taking logs, we get
y log x x log y
Differentiating w.r.t. x
1 dy 1 dy
y log x x log y 1
x dx y dx
dy x y
i.e., log x log y
dx y x
dy y log x x x log y y
i.e.,
dx y x
dy y x log y y
dx x y log x x
dy
Find , if,
dx
Task
y
x
1. x + y + 2a = 0
x
y
2. x – y + 2ax = 0
10.2 Logarithmic Differentiation Problems
The following problems illustrate the process of logarithmic differentiation. It is a means of
differentiating algebraically complicated functions or functions for which the ordinary rules of
differentiation do not apply. For example, in the problems that follow, you will be asked to
differentiate expressions where a variable is raised to a variable power. An example and two
common incorrect solutions are :
1. D x (2x 3) = (2x 3)x (2x 3) 1 (2x 3)x (2x 2)
and
2. D x (2x 3) = x (2x 3) (2)ln x
Both of these solutions are wrong because the ordinary rules of differentiation do not apply.
Logarithmic differentiation will provide a way to differentiate a function of this type. It requires
deft algebra skills and careful use of the following unpopular, but well-known, properties of
LOVELY PROFESSIONAL UNIVERSITY 271