Page 50 - DMTH201_Basic Mathematics-1

Unit 2: Trigonometric Functions-II

2. The cosine law may be used as follows: Notes
o
2
2
2
d = 72 + 50 – 2 (72)(50) cos(49 )
3. Solve for d and use calculator.
2
d = SQRT [72 + 50 – 2 (72)(50) cos(49 )]
o
2
(approximately) = 54.4 km

1. A triangle has sides equal to 4 m, 11 m and 8 m. Find its angles (round answers to 1
decimal place).
2. A ship leaves port at 1 pm traveling north at the speed of 30 miles/hour. At 3 pm, the
ship adjusts its course 20 degrees eastward. How far is the ship from the port at 4pm?
(round to the nearest unit).
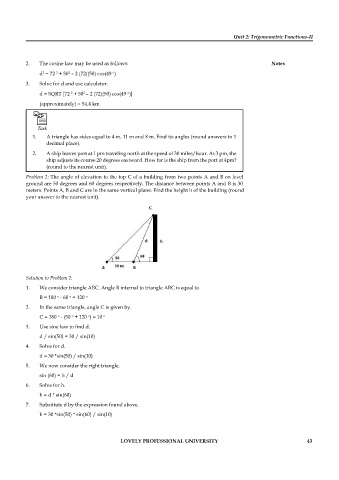
Problem 2: The angle of elevation to the top C of a building from two points A and B on level
ground are 50 degrees and 60 degrees respectively. The distance between points A and B is 30
meters. Points A, B and C are in the same vertical plane. Find the height h of the building (round
your answer to the nearest unit).

Solution to Problem 2:

1. We consider triangle ABC. Angle B internal to triangle ABC is equal to
B = 180 - 60 = 120
o
o
o
2. In the same triangle, angle C is given by.
o
o
o
o
C = 180 - (50 + 120 ) = 10
3. Use sine law to find d.

d / sin(50) = 30 / sin(10)
4. Solve for d.
d = 30 *sin(50) / sin(10)
5. We now consider the right triangle.
sin (60) = h / d
6. Solve for h.
h = d * sin(60)
7. Substitute d by the expression found above.
h = 30 *sin(50) * sin(60) / sin(10)

LOVELY PROFESSIONAL UNIVERSITY 43