Page 83 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes
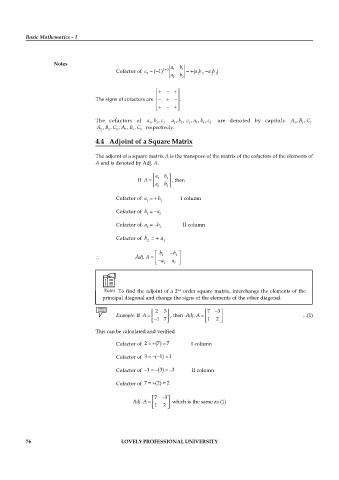
a 1 b 1
Cofactor of c 3 ( 1) 3 3 (a b a b )
2 1
1 2
a b
2 2
The signs of cofactors are .

,
,
,
,
,
The cofactors of a b c a b , c , a b c are denoted by capitals A B C
,
,
1 1 1 2 2 2 3 3 3 1 1 1
,
,
A , B C , A B C respectively.
,
2 2 2 3 3 3
4.4 Adjoint of a Square Matrix
The adjoint of a square matrix A is the transpose of the matrix of the cofactors of the elements of
A and is denoted by Adj. A.
a 1 b 1
If A , then
a b
2 2
Cofactor of a 1 b I column
2
Cofactor of b 1 a 2
Cofactor of a 2 b 1 II column
Cofactor of b 2 a 1

b b
Adj A 2 1
.
a 2 a 1

Notes To find the adjoint of a 2 order square matrix, interchange the elements of the
nd
principal diagonal and change the signs of the elements of the other diagonal.

2 3 7 3
Example: If A , then Adj A ...(1)
.
1 7 1 2
This can be calculated and verified

Cofactor of 2 (7) 7 I column

Cofactor of 3 ( 1) 1

Cofactor of 1 (3) 3 II column

Cofactor of 7 (2) 2

7 3
Adj . A which is the same as (1)
1 2

76 LOVELY PROFESSIONAL UNIVERSITY