Page 219 - DCAP507_SYSTEM_SOFTWARE

Unit 14: Canonic Systems

Three surfaces, the elliptic and hyperbolic paraboloids and the parabolic cylinder, do not have Notes
centers. In the case of these three, the point (x , y , z ) corresponds to their vertices. The elliptic
0 0 0
and hyperbolic paraboloids have a single vertex and the parabolic cylinder has a line of vertices.
In the case of a line of vertices, translation to any vertex will do. These vertices must be found by
some technique or procedure.
Determining the Orientation of the Canonic System

In the problem of translation to the canonic system origin we have noted that there may not be
a single point that we must translate to but instead we may have a range of points that we can
translate to (as in the case of a line of centers or a plane of centers). The same kind of situation
exists in the problem of finding the orientation of the canonic coordinate system. In some cases
there is a range of orientations that we can rotate to instead of just a single orientation. Consider
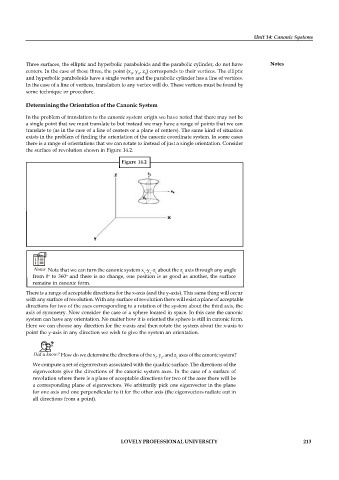
the surface of revolution shown in Figure 14.2.

Figure 14.2

Notes Note that we can turn the canonic system x -y -z about the z axis through any angle
c c c c
from 0 to 360 and there is no change, one position is as good as another, the surface
o
o
remains in canonic form.
There is a range of acceptable directions for the x-axis (and the y-axis). This same thing will occur
with any surface of revolution. With any surface of revolution there will exist a plane of acceptable
directions for two of the axes corresponding to a rotation of the system about the third axis, the
axis of symmetry. Now consider the case of a sphere located in space. In this case the canonic
system can have any orientation. No matter how it is oriented the sphere is still in canonic form.
Here we can choose any direction for the x-axis and then rotate the system about the x-axis to
point the y-axis in any direction we wish to give the system an orientation.

Did u know? How do we determine the directions of the x , y , and z axes of the canonic system?
c c c
We compute a set of eigenvectors associated with the quadric surface. The directions of the
eigenvectors give the directions of the canonic system axes. In the case of a surface of
revolution where there is a plane of acceptable directions for two of the axes there will be
a corresponding plane of eigenvectors. We arbitrarily pick one eigenvector in the plane
for one axis and one perpendicular to it for the other axis (the eigenvectors radiate out in
all directions from a point).

LOVELY PROFESSIONAL UNIVERSITY 213