Page 133 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

Notes Now, slope of the line l
m = tan
= tan ( 180° MPQ) = tan MPQ
MQ y y y y
= 2 1 2 1 .
MP x 1 x 2 x 2 x 1
Consequently, we see that in both the cases the slope m of the line through the points (x , y ) and
1 1
y y
(x , y ) is given by m 2 1 .
2 2
x 2 x 1
5.2.2 Conditions for Parallelism and Perpendicularity of Lines in terms
of their Slopes

In a coordinate plane, suppose that non-vertical lines l and l have slopes m and m , respectively.
1 2 1 2
Let their inclinations be and , respectively.
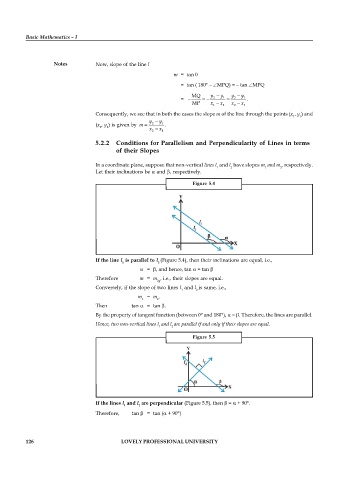
Figure 5.4

If the line l is parallel to l (Figure 5.4), then their inclinations are equal, i.e.,
1 2
= , and hence, tan = tan
Therefore m = m , i.e., their slopes are equal.
2g
Conversely, if the slope of two lines l and l is same, i.e.,
1 2
m = m .
1 2
Then tan = tan .
By the property of tangent function (between 0° and 180°), = . Therefore, the lines are parallel.
Hence, two non-vertical lines l and l are parallel if and only if their slopes are equal.
1 2
Figure 5.5

If the lines l and l are perpendicular (Figure 5.5), then = + 90°.
1 2
Therefore, tan = tan ( + 90°)

126 LOVELY PROFESSIONAL UNIVERSITY