Page 143 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

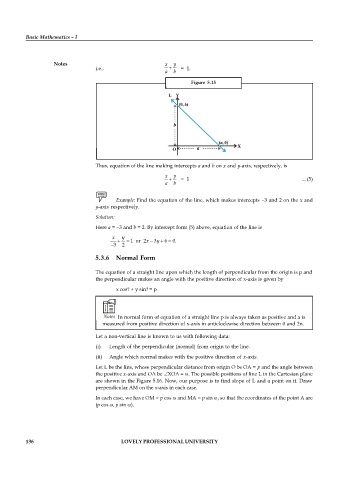
Notes x y
i.e., = 1.
a b
Figure 5.15

Thus, equation of the line making intercepts a and b on x and y-axis, respectively, is
x y
= 1 ... (5)
a b

Example: Find the equation of the line, which makes intercepts 3 and 2 on the x and
y-axis respectively.
Solution:
Here a = 3 and b = 2. By intercept form (5) above, equation of the line is

x y
1 or 2x 3y 6 0.
3 2
5.3.6 Normal Form

The equation of a straight line upon which the length of perpendicular from the origin is p and
the perpendicular makes an angle with the positive direction of x-axis is given by
x cos + y sin = p

Notes In normal form of equation of a straight line p is always taken as positive and a is
measured from positive direction of x-axis in anticlockwise direction between 0 and 2n.

Let a non-vertical line is known to us with following data:

(i) Length of the perpendicular (normal) from origin to the line.
(ii) Angle which normal makes with the positive direction of x-axis.
Let L be the line, whose perpendicular distance from origin O be OA = p and the angle between
the positive x-axis and OA be XOA = . The possible positions of line L in the Cartesian plane
are shown in the Figure 5.16. Now, our purpose is to find slope of L and a point on it. Draw
perpendicular AM on the x-axis in each case.

In each case, we have OM = p cos and MA = p sin , so that the coordinates of the point A are
(p cos , p sin ).

136 LOVELY PROFESSIONAL UNIVERSITY