Page 151 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

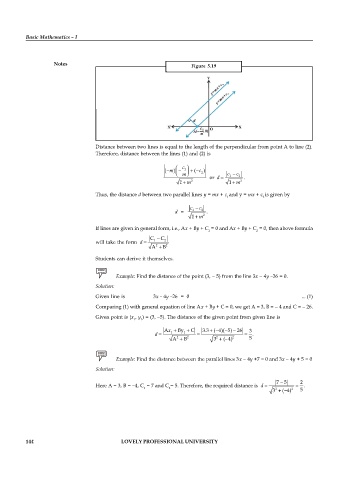
Notes Figure 5.19

Distance between two lines is equal to the length of the perpendicular from point A to line (2).
Therefore, distance between the lines (1) and (2) is

c
( m ) 1 ( c 2 )
m c 1 c 2
or d .
1 m 2 1 m 2
Thus, the distance d between two parallel lines y = mx + c and y = mx + c is given by
1 2
c c
d = 1 2 .
1 m 2

If lines are given in general form, i.e., Ax + By + C = 0 and Ax + By + C = 0, then above formula
1 2
C 1 C 2
will take the form d
A 2 B 2
Students can derive it themselves.

Example: Find the distance of the point (3, 5) from the line 3x 4y –26 = 0.
Solution:
Given line is 3x – 4y –26 = 0 ... (1)

Comparing (1) with general equation of line Ax + By + C = 0, we get A = 3, B = 4 and C = 26.
Given point is (x , y ) = (3, 5). The distance of the given point from given line is
1 1
Ax By C 3.3 ( 4)( 5) 26 3
d 1 1 .
A 2 B 2 3 2 ( 4) 2 5

Example: Find the distance between the parallel lines 3x 4y +7 = 0 and 3x 4y + 5 = 0
Solution:

7 5 2
Here A = 3, B = 4, C = 7 and C = 5. Therefore, the required distance is d .
1 2 2 2
3 ( 4) 5

144 LOVELY PROFESSIONAL UNIVERSITY