Page 321 - DMTH201_Basic Mathematics-1

Basic Mathematics – I

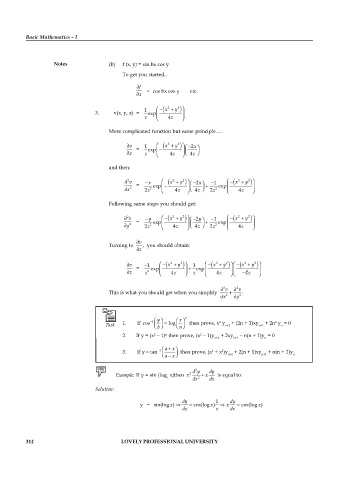
Notes (b) f (x, y) = sin hx cos y
To get you started..

f
= cos hx cos y etc.
x

1 x 2 y 2
3. v(x, y, z) = exp
z 4z
More complicated function but same principle….

v 1 x 2 y 2 2x
= exp
x z 4z 4z
and then:

2 2 2 2 2
v x x y 2x 1 x y
= exp exp
x 2 2z 2 4z 4z 2z 2 4z
Following same steps you should get:

2 2 2 2 2
v y x y 2y 1 x y
= exp exp
y 2 2z 2 4z 4z 2z 2 4z

v
Turning to , you should obtain:
z

v 1 x 2 y 2 1 x 2 y 2 x 2 y 2
= exp exp
2
z z 2 4z z 4z 4z
2 v 2 v
This is what you should get when you simplify .
x 2 y 2

1 y x n 2 2
Task 1. If cos b log n then prove, x y + (2n + 1)xy + 2n y = 0
n
n+2
n+1
n
2
2
2. If y = (x 1) then prove, (x 1)y + 2xy n(n + 1)y = 0
n+2 n+1 n
1 a x
2
3. If y tan then prove, (a + x )y + 2(n + 1)xy + n(n + 1)y
2
a x n+2 n+1 n
2
d y dy
Example: If y = sin (log x)then x 2 x is equal to:
e 2
dx dx
Solution:
dy 1 dy
x
y = sin(log )x cos(log ) x cos(log )
x
dx x dx
314 LOVELY PROFESSIONAL UNIVERSITY