Page 93 - DMTH201_Basic Mathematics-1
P. 93
Basic Mathematics – I
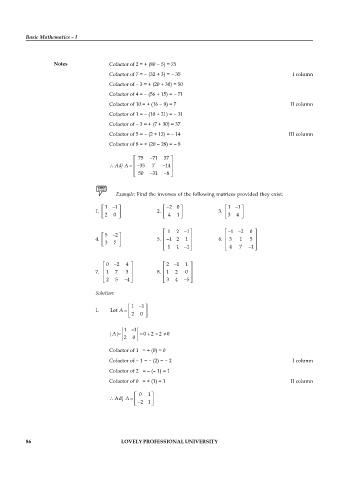
Notes Cofactor of 2 = + (80 5) = 75
Cofactor of 7 = (32 + 3) = 35 I column
Cofactor of 3 = + (20 + 30) = 50
Cofactor of 4 = (56 + 15) = 71
Cofactor of 10 = + (16 9) = 7 II column
Cofactor of 1 = (10 + 21) = 31
Cofactor of 3 = + (7 + 30) = 37
Cofactor of 5 = (2 + 12) = 14 III column
Cofactor of 8 = + (20 28) = 8
75 71 37
Adj A 35 7 14
50 31 8
Example: Find the inverses of the following matrices provided they exist:
1 1 2 0 1 1
1. 2. 3.
2 0 4 1 3 4
1 2 1 1 2 0
5 2
4. 5. 1 2 1 6. 3 1 5
3 7
1 1 1 4 7 1
0 2 4 2 1 1
7. 1 7 3 8. 1 2 0
2 5 4 3 4 5
Solution:
1 1
1. Let A
2 0
1 1
| | 0 2 2 0
A
2 0
Cofactor of 1 = + (0) = 0
Cofactor of 1 = (2) = 2 I column
Cofactor of 2 = ( 1) = 1
Cofactor of 0 = + (1) = 1 II column
0 1
Adj A
2 1
86 LOVELY PROFESSIONAL UNIVERSITY