Page 101 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes Solution:
Direct Method
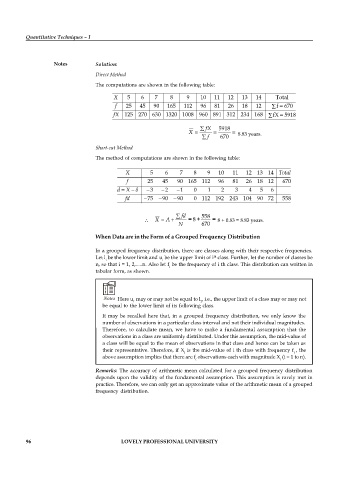
The computations are shown in the following table:

5 6 7 8 9 10 11 12 13 14 Total
25 45 90 165 112 96 81 26 18 12 f 670
125 270 630 1320 1008 960 891 312 234 168 fX 5918

5918
8.83 years.
670
Short-cut Method
The method of computations are shown in the following table:

5 6 7 8 9 10 11 12 13 14
25 45 90 165 112 96 81 26 18 12 670
3 2 1 0 1 2 3 4 5 6
75 90 90 0 112 192 243 104 90 72 558

558
= 8 + = 8 + 0.83 = 8.83 years.
670
When Data are in the Form of a Grouped Frequency Distribution

In a grouped frequency distribution, there are classes along with their respective frequencies.
th
Let l be the lower limit and u be the upper limit of i class. Further, let the number of classes be
i i
n, so that i = 1, 2,.....n. Also let f be the frequency of i th class. This distribution can written in
i
tabular form, as shown.

Notes Here u may or may not be equal to l , i.e., the upper limit of a class may or may not
1 2
be equal to the lower limit of its following class.
It may be recalled here that, in a grouped frequency distribution, we only know the
number of observations in a particular class interval and not their individual magnitudes.
Therefore, to calculate mean, we have to make a fundamental assumption that the
observations in a class are uniformly distributed. Under this assumption, the mid-value of
a class will be equal to the mean of observations in that class and hence can be taken as
their representative. Therefore, if X is the mid-value of i th class with frequency f , the
i i
above assumption implies that there are f observations each with magnitude X (i = 1 to n).
i i
Remarks: The accuracy of arithmetic mean calculated for a grouped frequency distribution
depends upon the validity of the fundamental assumption. This assumption is rarely met in
practice. Therefore, we can only get an approximate value of the arithmetic mean of a grouped
frequency distribution.

96 LOVELY PROFESSIONAL UNIVERSITY