Page 161 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes Solution:
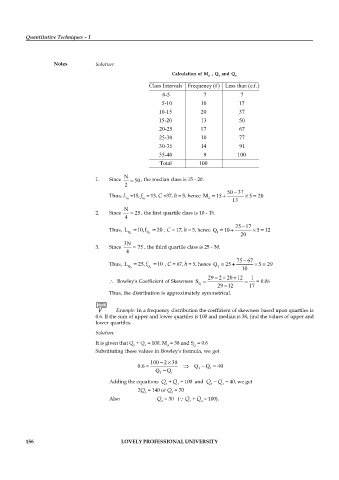
Calculation of M , Q and Q
d 1 3
Class Intervals Frequency (f ) Less than (c.f.)
0-5 7 7
5-10 10 17
10-15 20 37
15-20 13 50
20-25 17 67
25-30 10 77
30-35 14 91
35-40 9 100
Total 100

N
1. Since 50 , the median class is 15 - 20.
2
50 37
Thus, =15, = 13, =37, = 5, hence M 15 5 20
d
13
N
2. Since 25 , the first quartile class is 10 - 15.
4
25 17
Thus, L 10,f 20 = 17, = 5, hence Q 10 5 12
Q 1 1 Q 1
20
3N
3. Since 75 , the third quartile class is 25 - 30.
4
75 67
Thus, L 25,f 10 = 67, = 5, hence Q 25 5 29
Q 3 Q 3 3
10
29 2 20 12 1
Bowley's Coefficient of Skewness S = 0.06
Q
29 12 17
Thus, the distribution is approximately symmetrical.

Example: In a frequency distribution the coefficient of skewness based upon quartiles is
0.6. If the sum of upper and lower quartiles is 100 and median is 38, find the values of upper and
lower quartiles.
Solution:

It is given that Q + Q = 100, M = 38 and S = 0.6
3 1 d Q
Substituting these values in Bowley's formula, we get
100 2 38
0.6 Q 3 Q 1 40
Q 3 Q 1
Adding the equations + = 100 and = 40, we get
3 1 3 1
2 = 140 or = 70
3 3
Also = 30 ( + = 100).
1 1 3

156 LOVELY PROFESSIONAL UNIVERSITY