Page 258 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 12: Probability and Expected Value

2. Permutations of n objects taking r at a time: In terms of the example, considered above, Notes
now we have n persons to be seated on r chairs,
where r n .
n
Thus, P = n(n – 1)(n – 2) ...... [n – (r – 1)] = n(n – 1)(n – 2) ...... (n – r + 1).
r
On multiplication and division of the R.H.S. by (n - r)!, we get
n n 1 n 2 .... n r 1 n r ! n!
n
P r
n r ! (n r)!
3. Permutations of n objects taking r at a time when any object may be repeated any number
of times: Here, each of the r places can be filled in n ways. Therefore, total number of
permutations is nr.

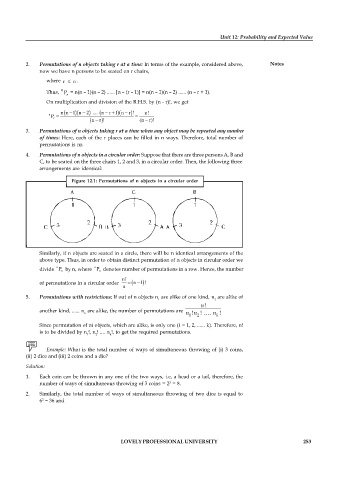
4. Permutations of n objects in a circular order: Suppose that there are three persons A, B and
C, to be seated on the three chairs 1, 2 and 3, in a circular order. Then, the following three
arrangements are identical:

Figure 12.1: Permutations of n objects in a circular order

Similarly, if n objects are seated in a circle, there will be n identical arrangements of the
above type. Thus, in order to obtain distinct permutation of n objects in circular order we
n
n
divide P by n, where P denotes number of permutations in a row. Hence, the number
n
n
n!
of permutations in a circular order n 1 !
n
5. Permutations with restrictions: If out of n objects n are alike of one kind, n are alike of
1 2
n!
another kind, ...... n are alike, the number of permutations are
k n !n ! .... n !
1 2 k
Since permutation of ni objects, which are alike, is only one (i = 1, 2, ...... k). Therefore, n!
is to be divided by n !, n ! .... n !, to get the required permutations.
1 2 k
Example: What is the total number of ways of simultaneous throwing of (i) 3 coins,
(ii) 2 dice and (iii) 2 coins and a die?
Solution:
1. Each coin can be thrown in any one of the two ways, i.e, a head or a tail, therefore, the
3
number of ways of simultaneous throwing of 3 coins = 2 = 8.
2. Similarly, the total number of ways of simultaneous throwing of two dice is equal to
6 = 36 and
2

LOVELY PROFESSIONAL UNIVERSITY 253