Page 71 - DMGT209_QUANTITATIVE_TECHNIQUES_II

Quantitative Techniques-II

Notes
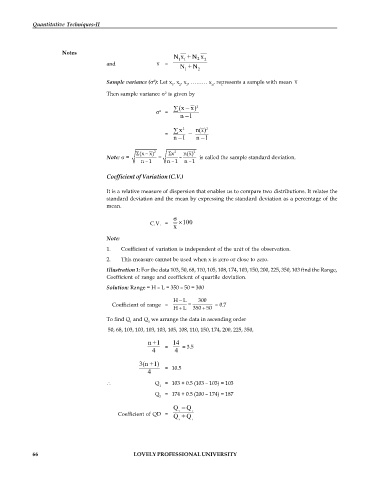
N x + N 2 x
1 1 2
and x =
N + N
1 2
2
Sample variance (s ): Let x , x , x , ……… x , represents a sample with mean x
1 2 3 n
Then sample variance s is given by
2

 (x x) 2
s 2 =

n 1
 x 2 n( x) 2


=

n 1 n 1

 (x – x) 2  x 2 n(x) 2
Note: s =  – is called the sample standard deviation.
n – 1 n – 1 n – 1
Coefficient of Variation (C.V.)
It is a relative measure of dispersion that enables us to compare two distributions. It relates the
standard deviation and the mean by expressing the standard deviation as a percentage of the
mean.

σ
C.V. =  100
x
Note:
1. Coefficient of variation is independent of the unit of the observation.
2. This measure cannot be used when x is zero or close to zero.
Illustration 1: For the data 103, 50, 68, 110, 105, 108, 174, 103, 150, 200, 225, 350, 103 find the Range,
Coefficient of range and coefficient of quartile deviation.
Solution: Range = H – L = 350 – 50 = 300

H L 300

Coefficient of range =  = 0.7
H L 350 50


To find Q and Q we arrange the data in ascending order
1 3
50, 68, 103, 103, 103, 103, 105, 108, 110, 150, 174, 200, 225, 350,
n +1 14
= = 3.5
4 4
3(n +1)
= 10.5
4
 Q = 103 + 0.5 (103 – 103) = 103
1
Q = 174 + 0.5 (200 – 174) = 187
3
Q  Q
3 1
Coefficient of QD = Q +Q
3 1

66 LOVELY PROFESSIONAL UNIVERSITY