Page 102 - DMGT207_MANAGEMENT_OF_FINANCES

Unit 5: Cost of Capital

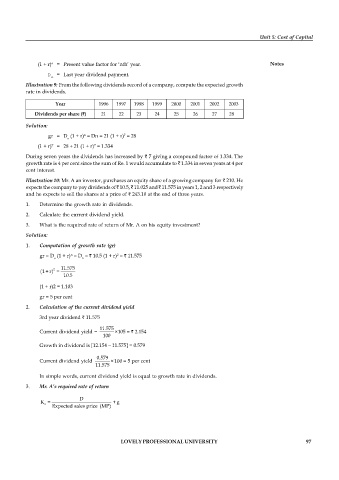
n
(1 + r) = Present value factor for ‘nth’ year. Notes
D = Last year dividend payment.
n
Illustration 9: From the following dividends record of a company, compute the expected growth
rate in dividends.
Year 1996 1997 1998 1999 2000 2001 2002 2003
Dividends per share ( ) 21 22 23 24 25 26 27 28

Solution:
gr = D (1 + r) = Dn = 21 (1 + r) = 28
7
n
o
7
(1 + r) = 28 ÷ 21 (1 + r) = 1.334
7
During seven years the dividends has increased by 7 giving a compound factor of 1.334. The
growth rate is 4 per cent since the sum of Re. 1 would accumulate to 1.334 in seven years at 4 per
cent interest.
Illustration 10: Mr. A an investor, purchases an equity share of a growing company for 210. He
expects the company to pay dividends of 10.5, 11.025 and 11.575 in years 1, 2 and 3 respectively
and he expects to sell the shares at a price of 243.10 at the end of three years.
1. Determine the growth rate in dividends.

2. Calculate the current dividend yield.
3. What is the required rate of return of Mr. A on his equity investment?
Solution:
1. Computation of growth rate (gr)

gr = D (1 + r) = D = 10.5 (1 + r) = 11.575
n
2
o n

2
1+ r = 11.575
10.5
(1 + r)2 = 1.103
gr = 5 per cent
2. Calculation of the current dividend yield
3rd year dividend 11.575

11.575
Current dividend yield = ×105 = 2.154
100
Growth in dividend is [12.154 – 11.575] = 0.579

0.579
Current dividend yield ×100 = 5 per cent
11.575
In simple words, current dividend yield is equal to growth rate in dividends.
3. Mr. A’s required rate of return

D
K = +g
e
Expected sales price (MP)

LOVELY PROFESSIONAL UNIVERSITY 97