Page 233 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

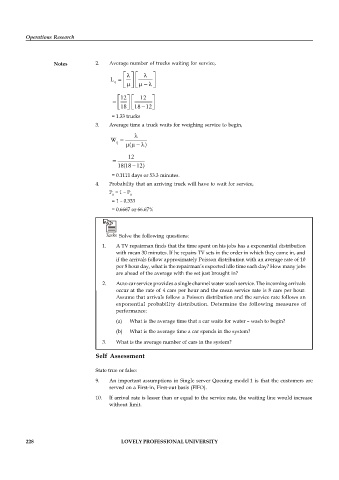
Notes 2. Average number of trucks waiting for service,

    
    

      
   

   
    
= 1.33 trucks
3. Average time a truck waits for weighing service to begin,


   



= 0.1111 days or 53.3 minutes.
4. Probability that an arriving truck will have to wait for service,
P = 1 – P
0 0
= 1 – 0.333
= 0.6667 or 66.67%

Tasks Solve the following questions:
1. A TV repairman finds that the time spent on his jobs has a exponential distribution
with mean 30 minutes. If he repairs TV sets in the order in which they come in, and
if the arrivals follow approximately Poisson distribution with an average rate of 10
per 8 hour day, what is the repairman’s expected idle time each day? How many jobs
are ahead of the average with the set just brought in?

2. Auto car service provides a single channel water wash service. The incoming arrivals
occur at the rate of 4 cars per hour and the mean service rate is 8 cars per hour.
Assume that arrivals follow a Poisson distribution and the service rate follows an
exponential probability distribution. Determine the following measures of
performance:
(a) What is the average time that a car waits for water – wash to begin?
(b) What is the average time a car spends in the system?
3. What is the average number of cars in the system?

Self Assessment

State true or false:
9. An important assumptions in Single server Queuing model 1 is that the customers are
served on a First-in, First-out basis (FIFO).

10. If arrival rate is lesser than or equal to the service rate, the waiting line would increase
without limit.

228 LOVELY PROFESSIONAL UNIVERSITY