Page 41 - DCOM303_DMGT504_OPERATION_RESEARCH

Operations Research

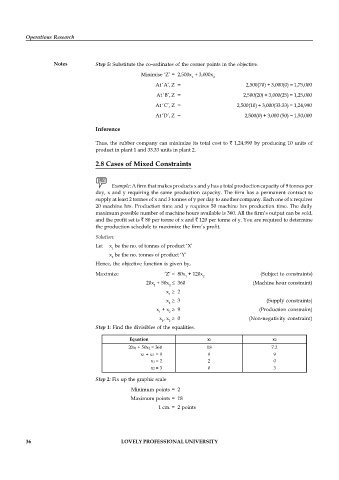
Notes Step 5: Substitute the co-ordinates of the corner points in the objective.
Minimise ‘Z’ = 2,500x + 3,000x
1 2
At ‘A’, Z = 2,500(70) + 3,000(0) = 1,75,000
At ‘B’, Z = 2,500(20) + 3,000(25) = 1,25,000

At ‘C’, Z = 2,500(10) + 3,000(33.33) = 1,24,990
At ‘D’, Z = 2,500(0) + 3,000 (50) = 1,50,000

Inference

Thus, the rubber company can minimize its total cost to ` 1,24,990 by producing 10 units of
product in plant 1 and 33.33 units in plant 2.

2.8 Cases of Mixed Constraints

Example: A firm that makes products x and y has a total production capacity of 9 tonnes per
day, x and y requiring the same production capacity. The firm has a permanent contract to
supply at least 2 tonnes of x and 3 tonnes of y per day to another company. Each one of x requires
20 machine hrs. Production time and y requires 50 machine hrs production time. The daily
maximum possible number of machine hours available is 360. All the firm’s output can be sold,
and the profit set is ` 80 per tonne of x and ` 120 per tonne of y. You are required to determine
the production schedule to maximize the firm’s profit.

Solution:
Let x be the no. of tonnes of product ‘X’
1
x be the no. tonnes of product ‘Y’
2
Hence, the objective function is given by,

Maximize ‘Z’ = 80x + 120x (Subject to constraints)
1 2
20x + 50x  360 (Machine hour constraint)
1 2
x  2
1
x  3 (Supply constraints)
2
x + x  9 (Production constraint)
1 2
x , x  0 (Non-negativity constraint)
1 2
Step 1: Find the divisibles of the equalities.
Equation x1 x2
20x 1 + 50x 2 = 360 18 7.2
x1 + x2 = 9 9 9
x 1 = 2 2 0
x2 = 3 0 3

Step 2: Fix up the graphic scale
Minimum points = 2
Maximum points = 18
1 cm. = 2 points

36 LOVELY PROFESSIONAL UNIVERSITY