Page 61 - DMGT513_DERIVATIVES_AND_RISK_MANAGEMENT

Derivatives & Risk Management

Notes The number of futures contract, which minimizes risk, is given by
NFC =(Q /Q )*Q ... (5.9)
F S FC
Where Q is the quantity (or units) of the underlying asset represented by each futures contract.
FC
Generally the hedge ratio is 1.0. In case of perfect hedging (absence of asset mismatch and
maturity mismatch), the hedge ratio should be one because the futures profit or loss matches the
spot profit or loss. In simple form, the Hedge Ratio (HR) is defined as the ratio of size of futures
contract position to the size of cash position (size of exposure). Size refers to the product of
number of contract with the quantity (units) of the asset (underlying) represented by the contact
(futures/spot).
If the objective of the hedger is to minimize risk, a hedger ratio of 1.0 is not necessarily optimal.
If hedgers wish to minimize the variance of their total positions, it may be optimal to use a
hedge ratio different from 1.0 when there is no liquid futures contract that matures later than the
expiration of the hedge. A strategy known as rolling the hedge forward is sometimes used.
The optimal hedge ratio is the product of the coefficient of correlation between the change in
spot price during a period of time equal to life of the hedge and change in futures price during
a period of time equal to life of the hedge and the ratio of the standard deviation of change in
spot price during a period of time equal to life of the hedge to the standard deviation of change
in futures price during a period of time equal to life of the hedge.

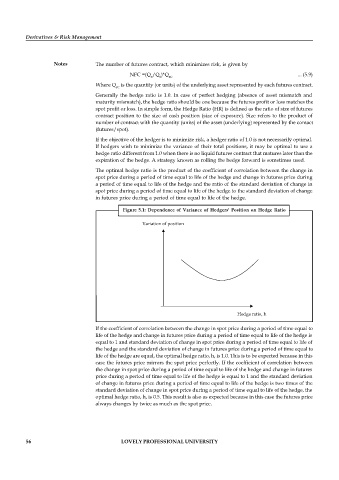
Figure 5.1: Dependence of Variance of Hedgers' Position on Hedge Ratio

Variation of position

Hedge ratio, h

If the coefficient of correlation between the change in spot price during a period of time equal to
life of the hedge and change in futures price during a period of time equal to life of the hedge is
equal to 1 and standard deviation of change in spot price during a period of time equal to life of
the hedge and the standard deviation of change in futures price during a period of time equal to
life of the hedge are equal, the optimal hedge ratio, h, is 1.0. This is to be expected because in this
case the futures price mirrors the spot price perfectly. If the coefficient of correlation between
the change in spot price during a period of time equal to life of the hedge and change in futures
price during a period of time equal to life of the hedge is equal to 1 and the standard deviation
of change in futures price during a period of time equal to life of the hedge is two times of the
standard deviation of change in spot price during a period of time equal to life of the hedge, the
optimal hedge ratio, h, is 0.5. This result is also as expected because in this case the futures price
always changes by twice as much as the spot price.

56 LOVELY PROFESSIONAL UNIVERSITY