Page 100 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 6: Measures of Central Tendency

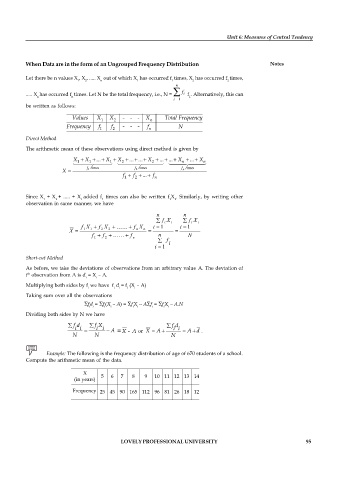
When Data are in the form of an Ungrouped Frequency Distribution Notes

Let there be n values X , X , ..... X out of which X has occurred f times, X has occurred f times,
1 2 n 1 1 2 2
n
f
..... X has occurred f times. Let N be the total frequency, i.e., N = i f . Alternatively, this can
n n 1
i 1
be written as follows:
Values X X - - - X Total Frequency
1 2 n
Frequency f f - - - f N
1 2 n
Direct Method
The arithmetic mean of these observations using direct method is given by
X 1 X 1 ... X 1 X 2 ... ... X 2 ... ... X n ... X n
  
f times f times n f times
X 1 2
f f ... f
1 2 n

Since X + X + ..... + X added f times can also be written f X . Similarly, by writing other
1 1 1 1 1 1
observation in same manner, we have
n n
f X i f X i
i
i
f X 1 f X 2  f X n i 1 i 1
2
n
1
X
f 1 f 2  f n n N
f
i
i 1
Short-cut Method
As before, we take the deviations of observations from an arbitrary value A. The deviation of
i observation from A is d = X – A.
th
i i
Multiplying both sides by f we have f d = f (X – A)
i i i i i
Taking sum over all the observations
= ( – ) = – = – .
Dividing both sides by N we have

= X - A or .

Example: The following is the frequency distribution of age of 670 students of a school.
Compute the arithmetic mean of the data.

X
5 6 7 8 9 10 11 12 13 14
(in years)
Frequency 25 45 90 165 112 96 81 26 18 12

LOVELY PROFESSIONAL UNIVERSITY 95