Page 102 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 6: Measures of Central Tendency

Notes
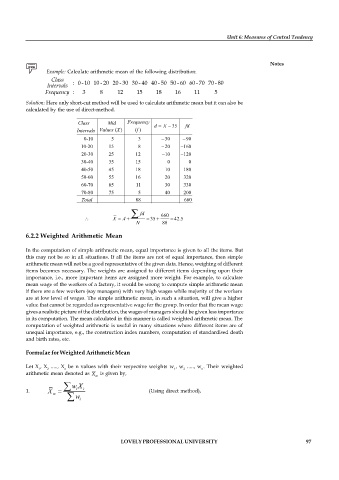
Example: Calculate arithmetic mean of the following distribution:
Class : 0 -10 10 - 20 20 - 30 30 - 40 40 - 50 50 - 60 60 -70 70 - 80
Intervals
Frequency : 3 8 12 15 18 16 11 5
Solution: Here only short-cut method will be used to calculate arithmetic mean but it can also be
calculated by the use of direct-method.
Class Mid Frequency
d X 35 fd
f
X
Intervals Values ( ) ( )
0-10 5 3 30 90
10-20 15 8 20 160
20-30 25 12 10 120
30-40 35 15 0 0
40-50 45 18 10 180
50-60 55 16 20 320
60-70 65 11 30 330
70-80 75 5 40 200
Total 88 660

fd 660
X A 35 42.5
N 88
6.2.2 Weighted Arithmetic Mean

In the computation of simple arithmetic mean, equal importance is given to all the items. But
this may not be so in all situations. If all the items are not of equal importance, then simple
arithmetic mean will not be a good representative of the given data. Hence, weighing of different
items becomes necessary. The weights are assigned to different items depending upon their
importance, i.e., more important items are assigned more weight. For example, to calculate
mean wage of the workers of a factory, it would be wrong to compute simple arithmetic mean
if there are a few workers (say managers) with very high wages while majority of the workers
are at low level of wages. The simple arithmetic mean, in such a situation, will give a higher
value that cannot be regarded as representative wage for the group. In order that the mean wage
gives a realistic picture of the distribution, the wages of managers should be given less importance
in its computation. The mean calculated in this manner is called weighted arithmetic mean. The
computation of weighted arithmetic is useful in many situations where different items are of
unequal importance, e.g., the construction index numbers, computation of standardised death
and birth rates, etc.

Formulae for Weighted Arithmetic Mean

Let X , X ....., X be n values with their respective weights w , w ....., w . Their weighted
1 2 n 1 2 n
arithmetic mean denoted as X is given by,
w
w X
1. X i i (Using direct method),
w
w
i

LOVELY PROFESSIONAL UNIVERSITY 97