Page 127 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I
P. 127
Quantitative Techniques – I
Notes Solution:
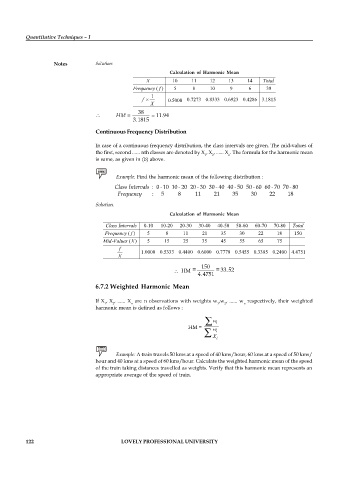
Calculation of Harmonic Mean
X 10 11 12 13 14 Total
Frequency ( ) 5 8 10 9 6 38
f
1
f 0.5000 0.7273 0.8333 0.6923 0.4286 3.1815
X
38
= = 11.94
3.1815
Continuous Frequency Distribution
In case of a continuous frequency distribution, the class intervals are given. The mid-values of
the first, second ...... nth classes are denoted by X , X , ...... X . The formula for the harmonic mean
1 2 n
is same, as given in (b) above.
Example: Find the harmonic mean of the following distribution :
Class Intervals : 0 -10 10 - 20 20 - 30 30 - 40 40 - 50 50 - 60 60 - 70 70 - 80
Frequency : 5 8 11 21 35 30 22 18
Solution.
Calculation of Harmonic Mean
Class Intervals 0-10 10-20 20-30 30-40 40-50 50-60 60-70 70-80 Total
f
Frequency ( ) 5 8 11 21 35 30 22 18 150
Mid -Values ( ) 5 15 25 35 45 55 65 75
X
f
1.0000 0.5333 0.4400 0.6000 0.7778 0.5455 0.3385 0.2400 4.4751
X
150
HM = = 33.52 Ans.
4.4751
6.7.2 Weighted Harmonic Mean
If X , X , ...... X are n observations with weights w ,w , ...... w respectively, their weighted
1 2 n 1 2 n
harmonic mean is defined as follows :
w i
HM = w
i
X i
Example: A train travels 50 kms at a speed of 40 kms/hour, 60 kms at a speed of 50 kms/
hour and 40 kms at a speed of 60 kms/hour. Calculate the weighted harmonic mean of the speed
of the train taking distances travelled as weights. Verify that this harmonic mean represents an
appropriate average of the speed of train.
122 LOVELY PROFESSIONAL UNIVERSITY