Page 122 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 6: Measures of Central Tendency

Notes
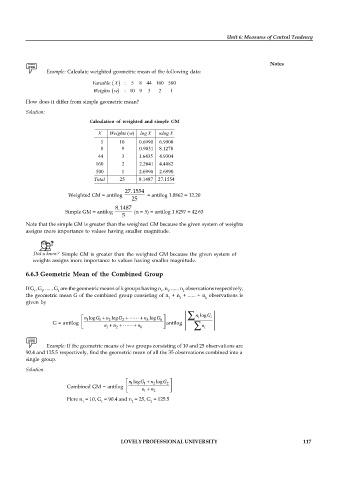
Example: Calculate weighted geometric mean of the following data:

Variable X : 5 8 44 160 500
Weights w : 10 9 3 2 1
How does it differ from simple geometric mean?
Solution:
Calculation of weighted and simple GM

w
X Weights ( ) log X wlog X
5 10 0.6990 6.9900
8 9 0.9031 8.1278
44 3 1.6435 4.9304
160 2 2.2041 4.4082
500 1 2.6990 2.6990
Total 25 8.1487 27.1554
27.1554
Weighted GM = antilog = antilog 1.0862 = 12.20
25
8.1487
Simple GM = antilog (n = 5) = antilog 1.6297 = 42.63
5
Note that the simple GM is greater than the weighted GM because the given system of weights
assigns more importance to values having smaller magnitude.

Did u know? Simple GM is greater than the weighted GM because the given system of
weights assigns more importance to values having smaller magnitude.

6.6.3 Geometric Mean of the Combined Group

If G , G , ...... G are the geometric means of k groups having n , n , ...... n observations respectively,
1 2 k 1 2 k
the geometric mean G of the combined group consisting of n + n + ...... + n observations is
1 2 k
given by
n 1 logG 1 n 2 logG 2  n k logG k n i logG i
G = antilog antilog
n n  n n
1 2 k i

Example: If the geometric means of two groups consisting of 10 and 25 observations are
90.4 and 125.5 respectively, find the geometric mean of all the 35 observations combined into a
single group.
Solution.

n 1 logG 1 n 2 logG 2
Combined GM = antilog
n 1 n 2
Here n = 10, G = 90.4 and n = 25, G = 125.5
1 1 2 2

LOVELY PROFESSIONAL UNIVERSITY 117