Page 118 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 6: Measures of Central Tendency

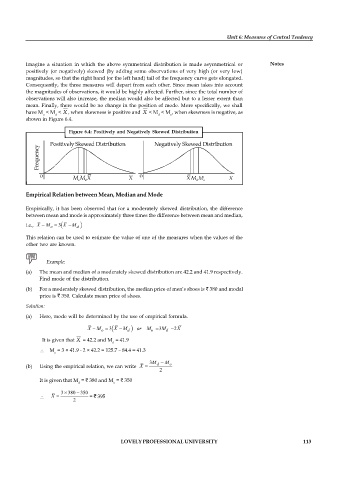
Imagine a situation in which the above symmetrical distribution is made asymmetrical or Notes
positively (or negatively) skewed (by adding some observations of very high (or very low)
magnitudes, so that the right hand (or the left hand) tail of the frequency curve gets elongated.
Consequently, the three measures will depart from each other. Since mean takes into account
the magnitudes of observations, it would be highly affected. Further, since the total number of
observations will also increase, the median would also be affected but to a lesser extent than
mean. Finally, there would be no change in the position of mode. More specifically, we shall
have M < M < X , when skewness is positive and X < M < M , when skewness is negative, as

o d d o
shown in Figure 6.4.
Figure 6.4: Positively and Negatively Skewed Distribution

Empirical Relation between Mean, Median and Mode

Empirically, it has been observed that for a moderately skewed distribution, the difference
between mean and mode is approximately three times the difference between mean and median,
i.e., X M o 3 X M d

This relation can be used to estimate the value of one of the measures when the values of the
other two are known.

Example:
(a) The mean and median of a moderately skewed distribution are 42.2 and 41.9 respectively.
Find mode of the distribution.
(b) For a moderately skewed distribution, the median price of men’s shoes is 380 and modal
price is 350. Calculate mean price of shoes.

Solution:
(a) Here, mode will be determined by the use of empirical formula.

X M o 3 X M d or M o 3M d 2X
It is given that X = 42.2 and M = 41.9
d
M = 3 × 41.9 - 2 × 42.2 = 125.7 – 84.4 = 41.3
o
3M d M o
(b) Using the empirical relation, we can write X
2
It is given that M = 380 and M = 350
d o
3 380 350
X = 395
2

LOVELY PROFESSIONAL UNIVERSITY 113