Page 193 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Quantitative Techniques – I

Notes
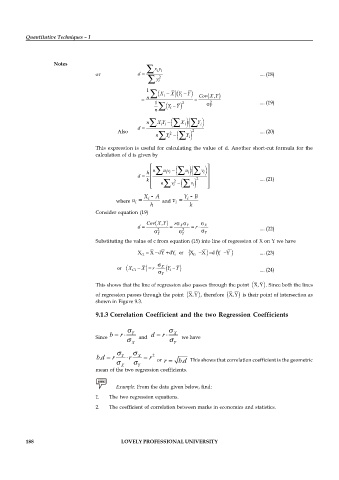
x y
i i
or d 2 .... (18)
y
i
1
X i X Y i Y
n Cov X ,Y
1 2 2 .... (19)
Y i Y Y
n
n X Y X Y
i i i i
d
Also 2 2 .... (20)
n Y i Y i
This expression is useful for calculating the value of d. Another short-cut formula for the
calculation of d is given by

n u v u v
h i i i i
d
k 2 2 .... (21)
n v i v i
X - A Y - B
where u = i and v = i
i
i
h k
Consider equation (19)
Cov X ,Y r X Y X
d r
2 2 .... (22)
Y Y Y
Substituting the value of c from equation (15) into line of regression of X on Y we have

X Ci X dY dY or X Ci X d Y i Y .... (23)
i
or X Ci X r X Y i Y .... (24)
Y
This shows that the line of regression also passes through the point X,Y . Since both the lines
of regression passes through the point X,Y , therefore X,Y is their point of intersection as
shown in Figure 9.3.
9.1.3 Correlation Coefficient and the two Regression Coefficients

b r Y d r X
Since and we have
X Y

. b d r Y r X r 2 or r . b d This shows that correlation coefficient is the geometric
X Y
mean of the two regression coefficients.

Example: From the data given below, find:
1. The two regression equations.

2. The coefficient of correlation between marks in economics and statistics.

188 LOVELY PROFESSIONAL UNIVERSITY