Page 196 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 9: Regression Analysis

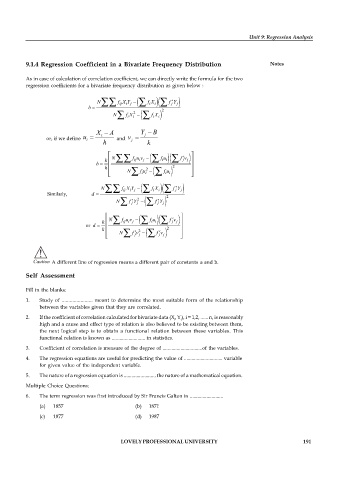
9.1.4 Regression Coefficient in a Bivariate Frequency Distribution Notes

As in case of calculation of correlation coefficient, we can directly write the formula for the two
regression coefficients for a bivariate frequency distribution as given below :

N f X Y f X f Y
ij i j i i j j
b
2
2
N f X i f X i
i
i
X A Y j B
or, if we define u i i and v j
h k
N f u v f u f v
k ij i j i i j j
b
h 2 2
N f u f u
i i
i i
N f X Y f X i f Y j
ij
i
i j
j
Similarly, d
2
N f Y j 2 f Y j
j
j
N f u v f u f v
h ij i j i i j j
or d
k 2 2
N f v f v
j j
j j
!
Caution A different line of regression means a different pair of constants a and b.
Self Assessment

Fill in the blanks:
1. Study of ....................... meant to determine the most suitable form of the relationship
between the variables given that they are correlated.
2. If the coefficient of correlation calculated for bivariate data (X, Y ), i = 1,2, ...... n, is reasonably
i i
high and a cause and effect type of relation is also believed to be existing between them,
the next logical step is to obtain a functional relation between these variables. This
functional relation is known as .......................... in statistics.
3. Coefficient of correlation is measure of the degree of ...............................of the variables.
4. The regression equations are useful for predicting the value of .............................. variable
for given value of the independent variable.
5. The nature of a regression equation is ......................... the nature of a mathematical equation.
Multiple Choice Questions:
6. The term regression was first introduced by Sir Francis Galton in ..........................

(a) 1857 (b) 1871
(c) 1877 (d) 1987

LOVELY PROFESSIONAL UNIVERSITY 191