Page 222 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I

Unit 10: Index Number

10.10.1 Chained Index Numbers Notes

The chain base index numbers, obtained above, are as such of not much use because these have
been computed with reference to a different base period and hence not comparable with each
other. To avoid this difficulty, these are required to be chained to a common base period. The
process of chaining is based upon the concept of circular test. The expression for chained index
for period ‘t’ with ‘0’ as base period, denoted as P Ch , can be written as
0t

Ch P 1 CB P 2 CB P t CB
P = × × L × ×100
0t
100 100 100
P CB ×P Ch P CB P CB
Ch t 0(t-1) Q P Ch = 1 ×L × t-1 ×100
or P 0t = 0(t-1) .... (2)
100 100 100
10.10.2 Conversion of Chain Base Index Number into Fixed Base Index
Number and vice-versa

FB
CB P 0t
P
We can write t FB 100
P 0 1
t
Chain Base Index
Fixed Base Index of current year
i.e., Number of current = ×100
Fixed Base Index of previous year .... (3)
year
Chain Base Index Fixed Base Index
×
Fixed Base Index of Current year of previous year
= .... (4)
of Current year 100
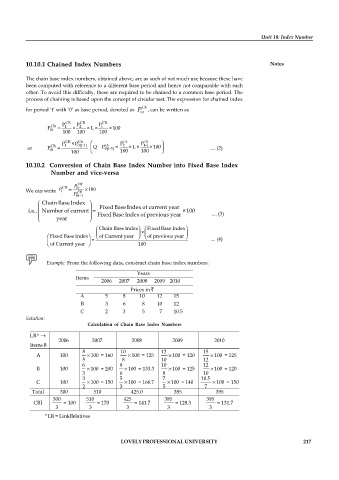
Example: From the following data, construct chain base index numbers:

Years
Items
2006 2007 2008 2009 2010
Prices in
A 5 8 10 12 15
B 3 6 8 10 12
C 2 3 5 7 10.5
Solution:
Calculation of Chain Base Index Numbers
LR*
2006 2007 2008 2009 2010
ItemsB
8 10 12 15
A 100 100 = 160 100 = 125 100 = 120 100 = 125
5 8 10 12
6 8 10 12
B 100 100 = 200 100 = 133.3 100 = 125 100 = 120
3 6 8 10
3 5 7 10.5
C 100 100 = 150 100 = 166.7 100 = 140 100 = 150
2 3 5 7
Total 300 510 425.0 385 395
300 510 425 385 395
CBI = 100 = 170 = 141.7 = 128.3 = 131.7
3 3 3 3 3
* LR LinkRelatives
=

LOVELY PROFESSIONAL UNIVERSITY 217