Page 235 - DCOM203_DMGT204_QUANTITATIVE_TECHNIQUES_I
P. 235
Quantitative Techniques – I
Notes The moving average of a group is always shown at the centre of its period. The process of
computing moving averages smoothens out the fluctuations in the time series data. It can
be shown that if the trend is linear and the oscillatory variations are regular, the moving
average with period equal to the period of oscillatory variations would completely
eliminate them. Further, the effect of random variations would get minimised because the
average of a number of observations must lie between the smallest and the largest
observation. It should be noted here that the larger is the period of moving average the
more would be the reduction in the effect of random component but more information is
lost at the two ends of data.
When the trend is non-linear, the moving averages would give biased rather than the
actual trend values.
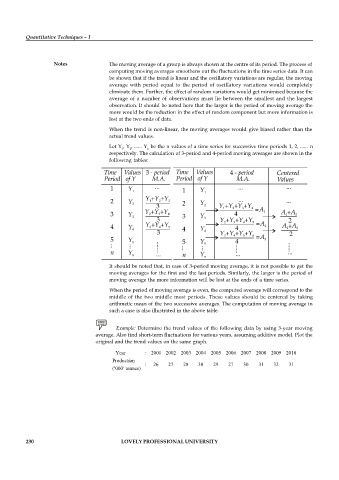
Let Y , Y , ...... Y be the n values of a time series for successive time periods 1, 2, ...... n
1 2 n
respectively. The calculation of 3-period and 4-period moving averages are shown in the
following tables:
It should be noted that, in case of 3-period moving average, it is not possible to get the
moving averages for the first and the last periods. Similarly, the larger is the period of
moving average the more information will be lost at the ends of a time series.
When the period of moving average is even, the computed average will correspond to the
middle of the two middle most periods. These values should be centered by taking
arithmetic mean of the two successive averages. The computation of moving average in
such a case is also illustrated in the above table.
Example: Determine the trend values of the following data by using 3-year moving
average. Also find short-term fluctuations for various years, assuming additive model. Plot the
original and the trend values on the same graph.
Year : 2001 2002 2003 2004 2005 2006 2007 2008 2009 2010
Production
: 26 27 28 30 29 27 30 31 32 31
('000' tonnes)
230 LOVELY PROFESSIONAL UNIVERSITY