Page 147 - DECO401_MICROECONOMIC_THEORY_ENGLISH

Microeconomic Theory

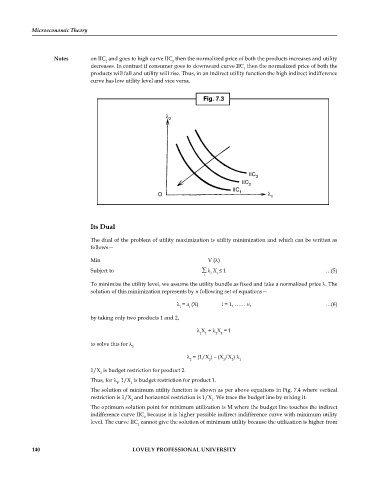
Notes on IIC and goes to high curve IIC then the normalized price of both the products increases and utility
2
3
decreases. In contrast if consumer goes to downward curve IIC then the normalized price of both the
1
products will fall and utility will rise. Thus, in an indirect utility function the high indirect indifference
curve has low utility level and vice versa.

Fig. 7.3

λ
2

IIC
3
IIC
2
IIC 1
O λ 1

Its Dual

The dual of the problem of utility maximization is utility minimization and which can be written as
follows—

Min V (λ)
Subject to ∑ λ X ≤ 1 …(5)

i i i
To minimize the utility level, we assume the utility bundle as fixed and take a normalized price λ. The
solution of this minimization represents by n following set of equations—

λ = a (X) i = 1, …… n, …(6)
i i
by taking only two products 1 and 2,
λ X + λ X = 1
1 1 2 2
to solve this for λ 2

λ = (1/X ) – (X /X ) λ 1
1
2
2
2
1/X is budget restriction for product 2.
2
Thus, for λ , 1/X is budget restriction for product 1.
1 1
The solution of minimum utility function is shown as per above equations in Fig. 7.4 where vertical
restriction is 1/X and horizontal restriction is 1/X . We trace the budget line by mixing it.
2 1
The optimum solution point for minimum utilization is M where the budget line touches the indirect
indifference curve IIC because it is higher possible indirect indifference curve with minimum utility
2
level. The curve IIC cannot give the solution of minimum utility because the utilization is higher from
1

140 LOVELY PROFESSIONAL UNIVERSITY