Page 323 - DECO403_MATHEMATICS_FOR_ECONOMISTS_ENGLISH

Mathematics for Economists

Note 3. Each industry produces only one product and there is no joint production of two products.
4. Total output of an industry is used as input for any other industry.
5. Production is done under rule of constant return.
6. Technical development is constant, it means that input coefficients are constant
7. Here in production no external austerity or improvidences are created

Leontief’s Static Input-Output Model – Open Model
Leontief’s Static Input-Output Model is based on the above assumptions. This can be understood
with an example. Suppose the economy is divided into three parts. Out of which agriculture and
industry are inter-trades and domestic part is expressed as last demand part.
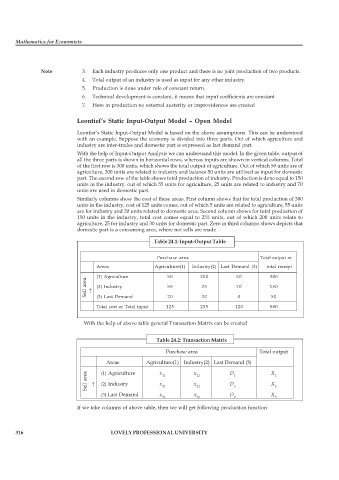
With the help of Input-Output Analysis we can understand this model. In the given table, output of
all the three parts is shown in horizontal rows, whereas inputs are shown in vertical columns. Total
of the first row is 300 units, which shows the total output of agriculture. Out of which 50 units are of
agriculture, 200 units are related to industry and balance 50 units are utilized as input for domestic
part. The second row of the table shows total production of industry. Production is done equal to 150
units in the industry, out of which 55 units for agriculture, 25 units are related to industry and 70
units are used in domestic part.
Similarly columns show the cost of these areas. First column shows that for total production of 300
units in the industry, cost of 125 units comes, out of which 5 units are related to agriculture, 55 units
are for industry and 20 units related to domestic area. Second column shows for total production of
150 units in the industry, total cost comes equal to 255 units, out of which 200 units relate to
agriculture, 25 for industry and 30 units for domestic part. Zero in third columns shows depicts that
domestic part is a consuming area, where not sells are made
Table 24.1: Input-Output Table

Purchase area Total output or
Areas Agriculture(1) Industry(2) Last Demand (3) total receipt
(1) Agriculture 50 200 50 300
Sell area → (2) Industry 55 25 70 150

20
50
(3) Last Demand
30
0
Total cost or Total input 125 255 120 500
With the help of above table general Transaction Matrix can be created

Table 24.2: Transaction Matrix
Purchase area Total output

Areas Agriculture(1) Industry(2) Last Demand (3)
Sell area → (1) Agriculture x 11 x 12 D 1 2 X 1 2

x
X
D
(2) Industry
x
21
22
(3) Last Demand x x D X
31 32 0 3
If we take columns of above table, then we will get following production function
316 LOVELY PROFESSIONAL UNIVERSITY