Page 324 - DECO403_MATHEMATICS_FOR_ECONOMISTS_ENGLISH

Unit 24: Input-Output Analysis

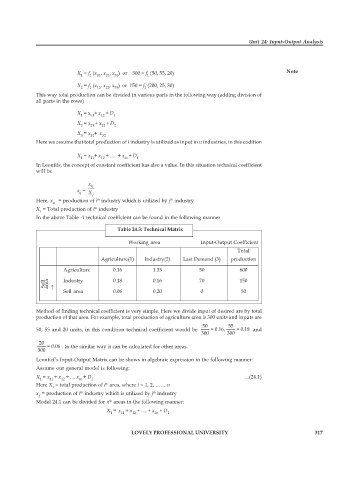
X = f (x , x , x ) or 300 = f (50, 55, 20) Note
11
21
1
1
31
1
X = f (x , x , x ) or 150 = f (200, 25, 30)
2
2
12
22
33
2
This way total production can be divided in various parts in the following way (adding division of
all parts in the rows)
X = x + x + D
1 11 12 1
X = x + x + D 2
2
21
22
X = x + x 32
31
3
Here we assume that total production of i industry is utilized as input in n industries, in this codition
X = x + x + ..... + x + D 1
12
11
in
1
In Leontifs, the concept of constant coefficient has also a value. In this situation technical coefficient
will be
x ij
a = X j
ij
Here, x = production of i industry which is utilized by j industry
th
th
11
X = Total production of i industry
th
1
In the above Table -1 technical coefficient can be found in the following manner
Table 24.3: Technical Matrix
Working area Input-Output Coefficient
Total
Agriculture(1) Industry(2) Last Demand (3) production
Agriculture 0.16 1.33 50 600
Sell area → Industry 0.18 0.16 70 150
Sell area 0.06 0.20 0 50

Method of finding technical coefficient is very simple. Here we divide input of desired are by total
production of that area. For example, total production of agriculture area is 300 units and inputs are
50 55
50, 55 and 20 units, in this condition technical coefficient would be =0.16, =0.18 and
300 300
20 =0.06 . In the similar way it can be calculated for other areas.
300
Leontief’s Input-Output Matrix can be shows in algebraic expression in the following manner:
Assume our general model is following:
X = x + x + .... x + D 1 ...(24.1)
12
in
1
11
Here X = total production of i area, where i = 1, 2, …… n
th
1
x = production of i industry which is utilized by j industry
th
th
ij
Model 24.1 can be divided for n areas in the following manner:
th
X = x + x + .... + x + D 1
1
11
12
1n
LOVELY PROFESSIONAL UNIVERSITY 317