Page 241 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 18: Methods—Simple Average of Price Relatives

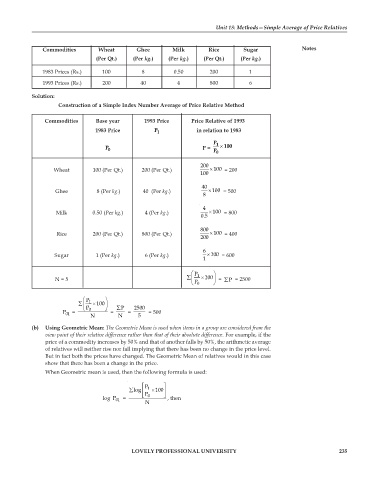
Commodities Wheat Ghee Milk Rice Sugar Notes
(Per Qt.) (Per kg.) (Per kg.) (Per Qt.) (Per kg.)

1983 Prices (Rs.) 100 8 0.50 200 1
1993 Prices (Rs.) 200 40 4 800 6

Solution:
Construction of a Simple Index Number Average of Price Relative Method

Commodities Base year 1993 Price Price Relative of 1993
1983 Price P 1 in relation to 1983

P 1
P 0 P = P 0 × 100

200
Wheat 100 (Per Qt.) 200 (Per Qt.) × 100 = 200
100
40
Ghee 8 (Per kg.) 40 (Per kg.) × 100 = 500
8
4
Milk 0.50 (Per kg.) 4 (Per kg.) × 100 = 800
0.5

800
Rice 200 (Per Qt.) 800 (Per Qt.) × 100 = 400
200
6
Sugar 1 (Per kg.) 6 (Per kg.) × 100 = 600
1

⎛ ⎞ P
N = 5 ∑ 1 × ⎜ 100 ⎟ = ∑P = 2500
⎝ P ⎠ 0

⎛ ⎞ P
∑ 1 × ⎜ 100 ⎟
⎝ P ⎠ 0 ∑ P 2500
P = N = N = 5 = 500
01
(b) Using Geometric Mean: The Geometric Mean is used when items in a group are considered from the
view point of their relative difference rather than that of their absolute difference. For example, if the
price of a commodity increases by 50% and that of another falls by 50%, the arithmetic average
of relatives will neither rise nor fall implying that there has been no change in the price level.
But in fact both the prices have changed. The Geometric Mean of relatives would in this case
show that there has been a change in the price.
When Geometric mean is used, then the following formula is used:

⎡ P ⎤
∑ log ⎢ 1 × 100 ⎥
log P 01 = ⎣ P 0 ⎦ , then
N

LOVELY PROFESSIONAL UNIVERSITY 235